Abstract
The electrochemical response of two biologically important phenazine derivatives was examined by using cyclic, differential pulse and square wave voltammetry in a wide pH range of 2.0 – 12.0. Cyclic voltammetry was employed for the determination of heterogeneous electron transfer rate constant and diffusion coefficient of the analytes. The value of electron transfer rate constant was used for evaluation of Gibbs free energy, enthalpy and entropy changes of redox reactions of the compounds. Differential pulse voltammetry was used for the determination of number of protons and electrons involved in the redox processes. Limits of detection and quantification were assessed by square wave voltammetry. The detailed pH dependent redox mechanisms of the compounds were proposed on the basis of experimental results which were further supported by theoretical calculations. These redox mechanistic pathways are expected to play a key role in understanding the necessary aspects of structure-activity relationships and exploring the hidden routes by which this class of compounds exert its biochemical actions.

This is an open access article distributed under the terms of the Creative Commons Attribution 4.0 License (CC BY, http://creativecommons.org/licenses/by/4.0/), which permits unrestricted reuse of the work in any medium, provided the original work is properly cited.
Phenazines have broad range applications in biology and chemistry.1 This class constitutes a large group of nitrogen containing heterocyclic compounds, the members of which differ in their chemical and physical properties on the basis of type and position of functional groups present. Naturally, phenazines are produced by limited genera of bacteria including Pseudomonas, Burkholderia and Brevibacterium.2 Starting from different parent materials, phenazines can be synthesized by various reactions including oxidative condensation, electrochemical reduction, reductive cyclization and photochemical reactions.
From the last 50 years, phenazines are the subject of extensive research investigations because of their broad range applications in pharmaceutical and clinical research.3 Such compounds have been reported for their wide variety of biological activities such as antitumor, anti-parasitic and antibiotic activities. Benzo [a] phenazine acts as efficient DNA intercalating ligand with antitumor activity in leukaemia and solid tumors.4 1-Hydroxyphenazine has been reported to have promising antifungal activity against various microbes such as aspergillus fumigatus and candida albicans.5 Macrolactone synthesized from benzo [a] phenazine shows good activity against mycobacterium tuberculosis.6 In general, natural and synthetic phenazines find useful applications against malaria7 and hepatitis C viral replication.8 The biological activity of phenazines is due to their redox reactions leading to the formation of toxic radicals.9 Different functional groups attached to phenazine determine their redox potential and solubility. The redox behavior and concomitant modulation of biological activities of phenazines by changing the nature and position of substituents attached to their aromatic rings have been reported by several research groups.10–12 Phenazines can alter cellular redox states and act as transporters of electrons to alternate terminal acceptors. Thus, phenazines serve as cell signals that adjust arrangement of gene expression.
Phenazines are extensively used as redox mediators and electrocatalysts due to their high selectivity and sensitivity as sensors, unique electrochemical properties and chromatic characteristics.13 Liu and co-workers have used N-methyl phenazine methosulfate as electron transfer mediator in the design of highly sensitive biosensors for hydrogen peroxide, lactose and glucose detection.14 Similarly, 3,7-bis(trimethylamino) phenothiazine has been employed as electrochemical sensor for the detection and quantification of hemoglobin.15 Phenazine-1-carboxylic acid acts as electron shuttle in microbial fuel cell (MFC) for transferring electron in alkaline environment between organic matter and anode of MFC.16 Phenazines are used as precursor for the preparation of dyes which find application as photosensitive materials solar cells.17,18 In addition to these applications, phenazines can also be utilized as components of organic light emitting devices.19 Thus stimulated by the broad range applications, relation of biological activity of phenazines with their redox reactions, structural diversity and some special properties (high sensitivity, unique redox behavior etc.) associated with phenazines, we investigated the effect of substituent, pH, temperature and sweep rate on the electrochemical response of two biologically important phenazines, benzo [a] phenazine and 3-bromo benzo [a] phenazine by using cyclic, differential pulse and square wave voltammetry.
To date, the redox behavior of some phenazines has been studied at platinum and hanging mercury drop electrodes. In this connection, the electrochemical reduction of phenazines in acetonitrile, dimethylsulfoxide, dimethylformamide and some other organic solvents has been investigated.20,21 The electrochemical polymerization of phenazine derivatives and the effect of pH on rate constant of phenazines have also been documented in literature.22,23
The electrochemical polymerization of phenazines depends not only on applied potential but also on the pH of the of the medium.24,25 Therefore, we investigated two unexplored phenazines (benzo[a]phenazine and 3-bromo benzo[a]phenazine abbreviated as BP and BBP) in a wide pH range and evaluated various useful kinetic, analytical and thermodynamic parameters of their redox reactions. The compounds BP and BBP were selected due to their robust electrochemical response in media of different pH. For offering convincing explanation to the experimental findings, computational chemistry was used to identify the most favorable oxidizable and reducible electrophores in the structures of the selected compounds.
Experimental
Materials and reagents
Benzo[a]phenazine and 3-bromo benzo[a] were kindly provided by Prof. Dr. Amin Badshah. Analytical grade ethanol was used as solvent for the preparation of 1 mM stock solutions of the compounds. Fresh working solutions of BP and BBP were prepared in 50% ethanol and 50% supporting electrolytes. Britton-Robinson buffer26 of pH range 2.0–12.0 was employed as supporting electrolyte.
Equipment and measurements
All voltammetric experiments were performed with μAutolab running with GPES 4.9 software Eco-Chemie, The Netherlands. Glassy carbon electrode with apparent surface area of 0.071 cm2 was used as working electrode. A Pt wire and Ag/AgCl (3 M KCl) electrode were used as counter and reference electrodes. Before every electrochemical experiment, dissolved oxygen was flushed out by purging nitrogen in the solution for 10 minutes. The surface of working electrode was polished with diamond spray (particle size 1 μm) followed by thorough rinsing with distilled water. Differential pulse and square wave voltammetric experiments were carried out at scan rate 5 and 100 mV s−1 respectively. The experimental conditions were 20 Hz frequency and 5 mV potential increments in square wave voltammetry. The temperature was maintained by immersing the measurement cell in water circulating bath (IRMECO I-2400 GmbH Germany).
Results and Discussion
Cyclic voltammetry of BP and BBP
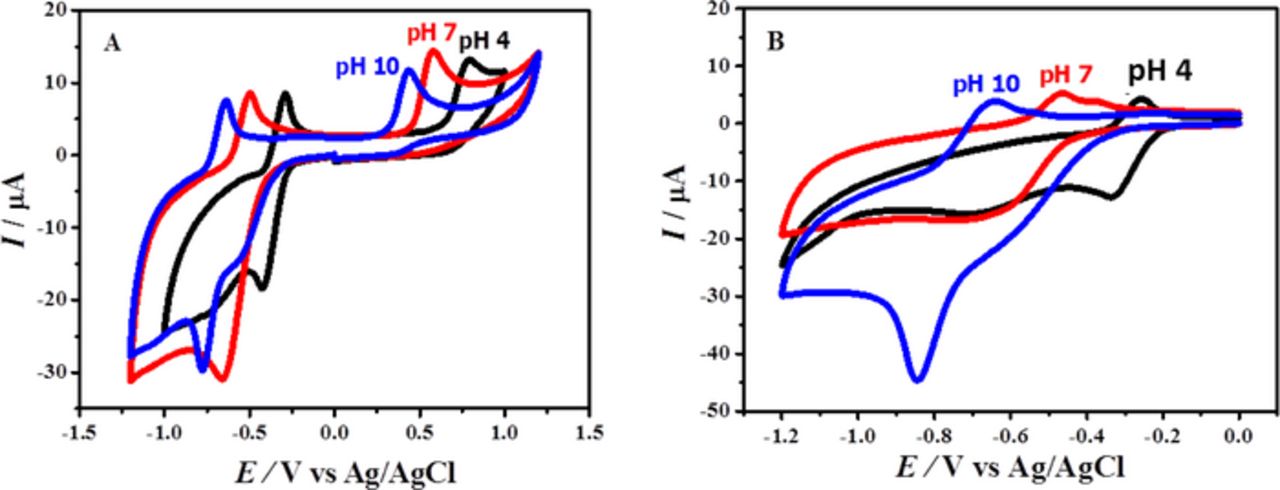
Electrochemical response of the compounds was first investigated by cyclic voltammetry (CV) in acidic, neutral and basic media to get a general picture of their redox behavior. While scanning the potential from zero to negative going direction, a prominent and stable cathodic signal was noticed in the forward scan of CVs (Fig. 1) of BP and BBP. An oxidation peak appeared in the reverse scan of cyclic voltammograms of both compounds. However, in the positive potential domain of GCE, only compound BP registered an oxidation signal. An observation of Fig. 1 reveals that the cyclic voltammetric signatures of the compounds are reversible but actually both the oxidation and reduction processes are irreversible in nature as evidenced by shift in peak potential with increase in scan rate, value of (Epc – Epa)/n greater than 212 mV and Ipc/Ipa ≠ 1. The irreversible reduction of phenazine has also been reported in literature.27
Figure 1. Cyclic voltammograms of 0.5 mM (A) BP and (B) BBP obtained in acidic, neutral and alkaline conditions at 100 mVs−1.
Cyclic voltammetry was also used to examine the influence of pH on the redox properties of phenazines. The CVs presented in Fig. 1 demonstrate the pH dependent behavior of all signals. The shift of oxidation peaks to less positive potentials with increasing pH indicates easier oxidation process in alkaline conditions. As the signals of BP and BBP were found sharp and well defined in media of pH 7 and 10, so, most of the voltammetric investigations were conducted in solutions of these selected pH conditions.
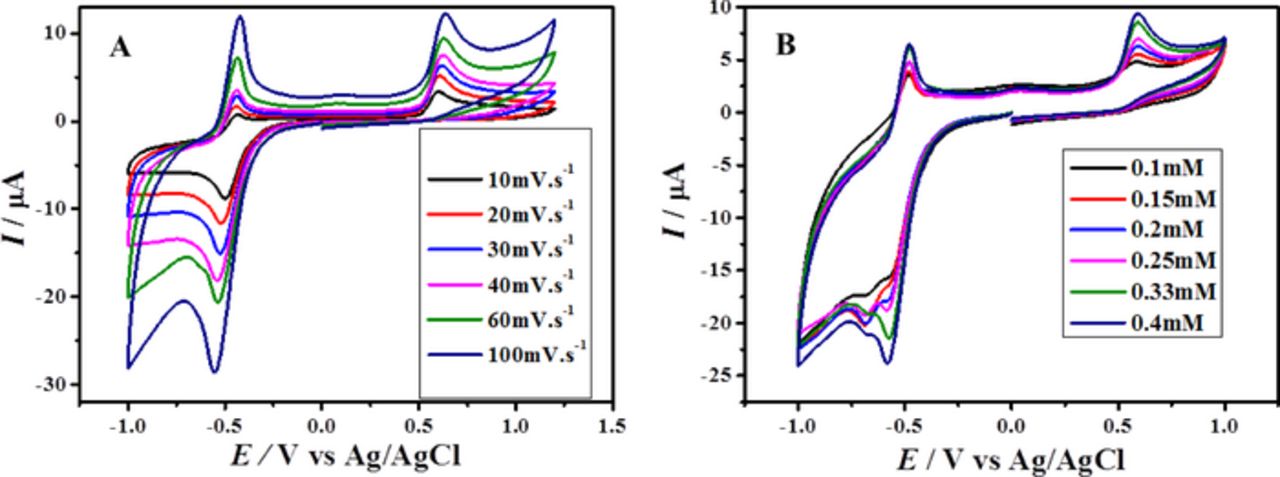
For the determination of diffusion coefficient (D), the effect of sweep rate on peak current (Ip) (Fig. 2A) was monitored by cyclic voltammetry. Peak current was plotted against the square root of scan rate and diffusion coefficient was calculated using Randles Sevcik equation.28 According to Stokes-Einstein equation, the smaller molecule (BP) should have higher D than comparatively larger molecule (BBP) but the opposite trend of D values listed in Table I can be related to the greater solvation number of more polar BP (having high dipole moment, see computational section) than BBP.29 The linearity of the plots between peak current and square root of scan rate suggests that the redox processes of the compounds and their reduced products are diffusion controlled.29–31
Table I. Parameters of BP and BBP obtained from electrochemical measurements.
| Peaks | W1/2/ mV | αn | α | n | D / cm2s−1 |
|---|---|---|---|---|---|
| Reduction peak of BP | 98 | 0.92 | 0.46 | 2 | 6.03(±027) × 10−6 |
| Oxidation peak of the reduced product of BP | 102 | 0.90 | 0.45 | 2 | — |
| Oxidation peak of BP | 90 | 1 | 0.5 | 2 | — |
| Reduction peak of BBP | 98 | 0.94 | 0.47 | 2 | 8.42(±032) × 10−6 |
| Oxidation peak of the reduced product of BBP | 100 | 0.92 | 0.46 | 2 | — |
Figure 2. (A) CVs of 0.5 mM BP at different scan rates and (B) cyclic voltammograms of different concentrations of BP at 100 mVs−1.
Cyclic voltammetry was also employed for the evaluation of heterogeneous electron transfer rate constant (ks). The peak current increased linearly with increase in concentration as expected. Interestingly, the single reduction peak of 0.5 mM BP split into two signals at lower concentration as clearly seen in Fig. 2B. The ks for both compounds was assessed from the slope of Ip versus concentration plot by using Reinmuth expression,28 Ip = nFAksC. The values of rate constant for reduction processes of BP at pH 7 and BBP at pH 10 were found to be 1.32 × 10−3 and 9.55 × 10−4 cm s−1 respectively. These values falling outside the range of a reversible redox process offer another evidence to support our attribution of irreversible reduction of BP and BBP.
Thermodynamic and kinetic parameters of BP and BBP
The effect of temperature on the oxidation and reduction signals of both compounds was investigated for the determination of enthalpy (ΔH) and entropy (ΔS) changes of electron transfer reactions. The peak current of the selected phenazine derivatives increased with rise in temperature due to the possible decrease in viscosity of the solution and consequent rapid diffusion of the analyte to the electrode surface. The values of ΔH and ΔS were evaluated from the slope and intercept of 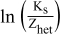 versus 1/T plots using equation 1.32,33
versus 1/T plots using equation 1.32,33
![Equation ([1])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn1.jpg)
where Zhet denotes collision number of a heterogeneous electron transfer process, which was calculated by using the following expression.34,35
![Equation ([2])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn2.jpg)
where M is molar mass of the compound and all other terms have their usual meanings.
The Gibbs free energy (ΔG) was assessed at different temperatures by applying equation 3.
![Equation ([3])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn3.jpg)
The slope of Arrhenius type plot of log ks versus 1/T was used for the determination of apparent energy of activation Ea using Einstein equation.35,36 The values of thermodynamic parameters are presented in Table II. The positive value of Gibbs free energy and enthalpy change reveal the non-spontaneous and endergonic nature of redox processes. The positive entropy change of electro-reduction of BP indicates entropy driven nature of reduction process while more ordered products formation as a result of all other reactions (reduction of BBP, oxidation of BP and its reduced product) are witnessed by the negative values of entropy changes.
Table II. Thermodynamic and kinetic parameters of the redox processes of BP and BBP.
| Nature of signals | pH | Ea (kJ mol−1) | ΔG (kJ mol−1) | ΔH (kJ mol−1) | ΔS (J K−1 mol−1) |
|---|---|---|---|---|---|
| reduction peak of BP | 7 | 29.5 | 24.63 | 28.3 | 12.3 |
| oxidation peak of the reduced product of BP | 7 | 25.7 | 26.29 | 24.7 | −5.3 |
| reduction process of BBP | 10 | 17.7 | 24.87 | 16.2 | −28.5 |
| oxidation process of BBP | 10 | 21.2 | 26.60 | 19.8 | −21.8 |
Differential pulse voltammetry (DPV)
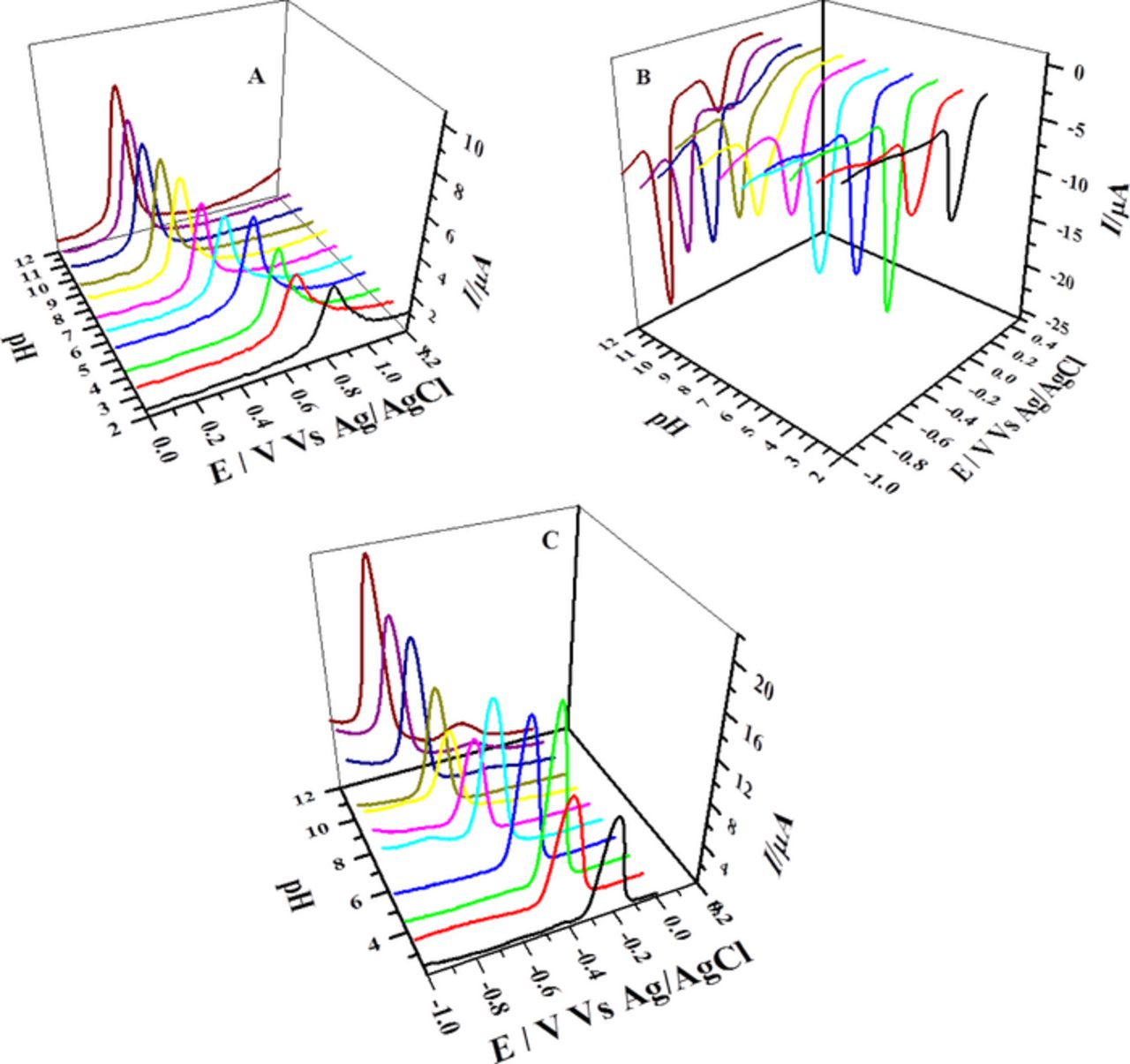
To examine the effect of pH, DPVs of 0.5 mM solutions of phenazines were obtained in the pH range 2.0–12.0 (Figs. 3 and 4). Like CV, stable and prominent oxidation and reduction signals were observed in differential pulse voltammograms of the analytes and their reduced products. However, quite different situation from CV was encountered when a new cathodic peak appeared under strongly alkaline conditions at potential lower than the prominent signal of both compounds as illustrated in Figs. 3B and 4A. The half peak width (W1/2) values obtained from DPVs were utilized for the determination of number of electrons "n" involved in all redox processes using equation 4.
![Equation ([4])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn4.jpg)
The values of W1/2, αn, electron transfer coefficient (α) and number of electrons involved in redox processes are listed in Table I.
Figure 3. Differential pulse voltammograms recorded in different pH media at 5 mVs−1 showing (A) oxidation (B) reduction and (C) oxidation of the reduced product of 0.5 mM BP.
Figure 4. Differential pulse voltammograms of 0.5 mM BBP recorded in different pH media at 5 mVs−1 showing (A) reduction of BBP and (B) oxidation of its reduced product.
DPVs demonstrated strong pH dependency of electrochemical response of the compounds and their redox products. Both oxidation and reduction signals shifted cathodically with increasing pH of the medium indicating proton coupled electron transfer reactions during oxidation and reduction of BP and BBP. Number of protons "P" involved in redox processes of the compounds was assessed from the slope of Ep versus pH plots using equation 5.33,37
![Equation ([5])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn5.jpg)
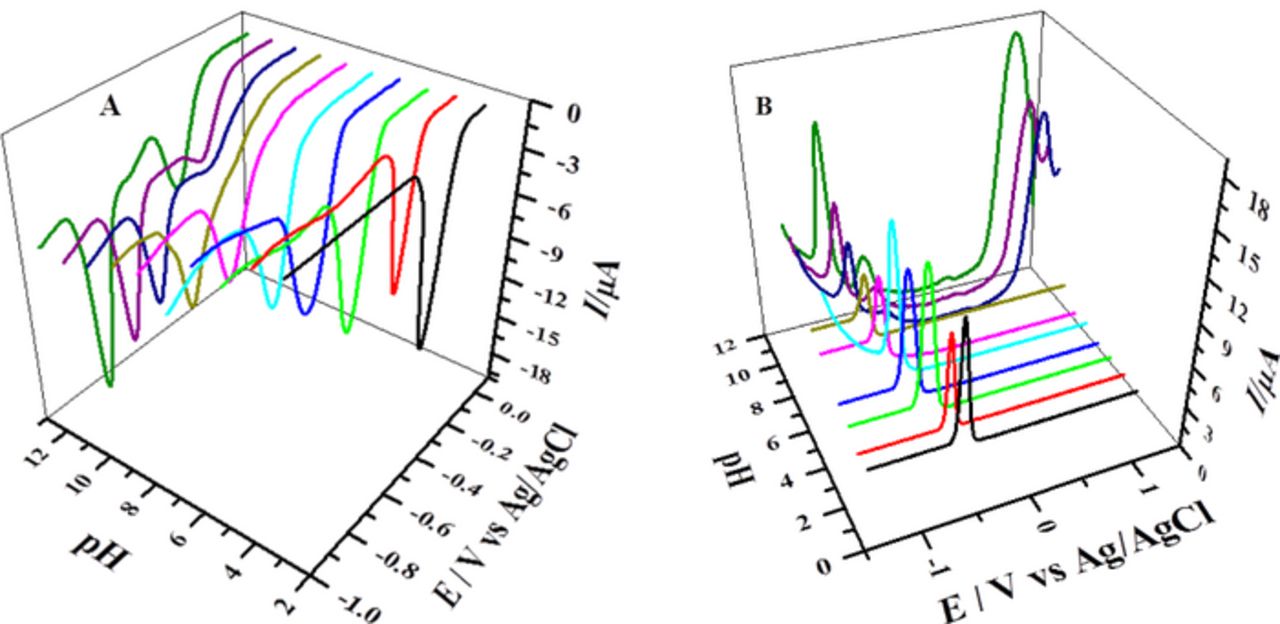
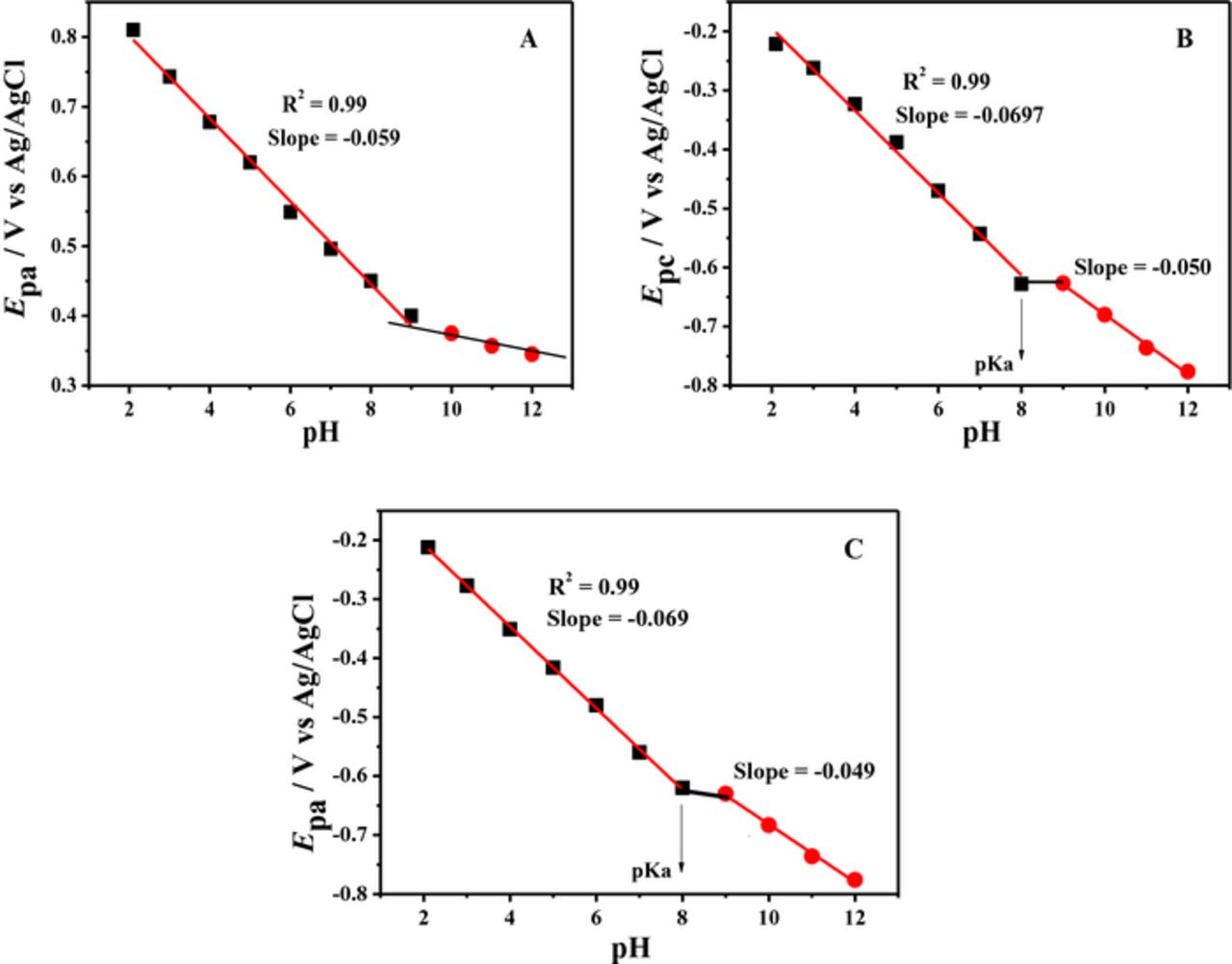
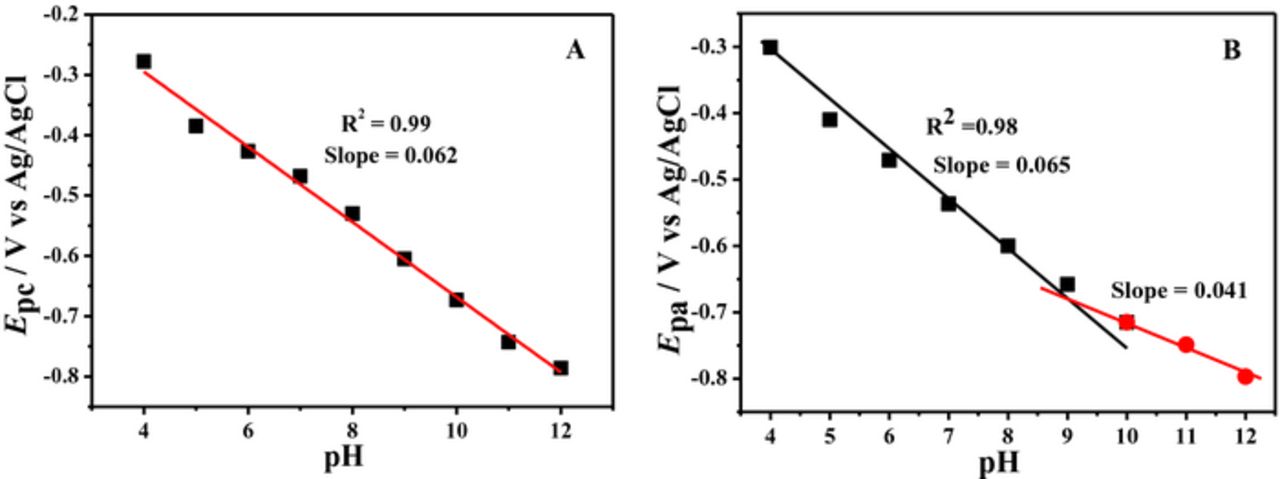
Acid-base dissociation constant, pKa was determined from the intersection point of the linear segments of Ep-pH plots as shown in Figs. 5 and 6. BP showed two pKa values of 8.0 and 9.0, while BBP demonstrated protonation-deprotonation at pH = 9.3. These values are comparable with the documented pKa = 8.2 of a phenazine derivative reported as neutral red.38 The parameters obtained from DPV helped in proposing the redox mechanism of the compounds.
Figure 5. Plots of (A) oxidation (B) Reduction and (C) Oxidation of reduced product of BP peak potentials versus pH.
Figure 6. Plots of (A) reduction peak potential of BBP and (B) oxidation peak potential of its reduced product versus pH.
Square wave voltammetry
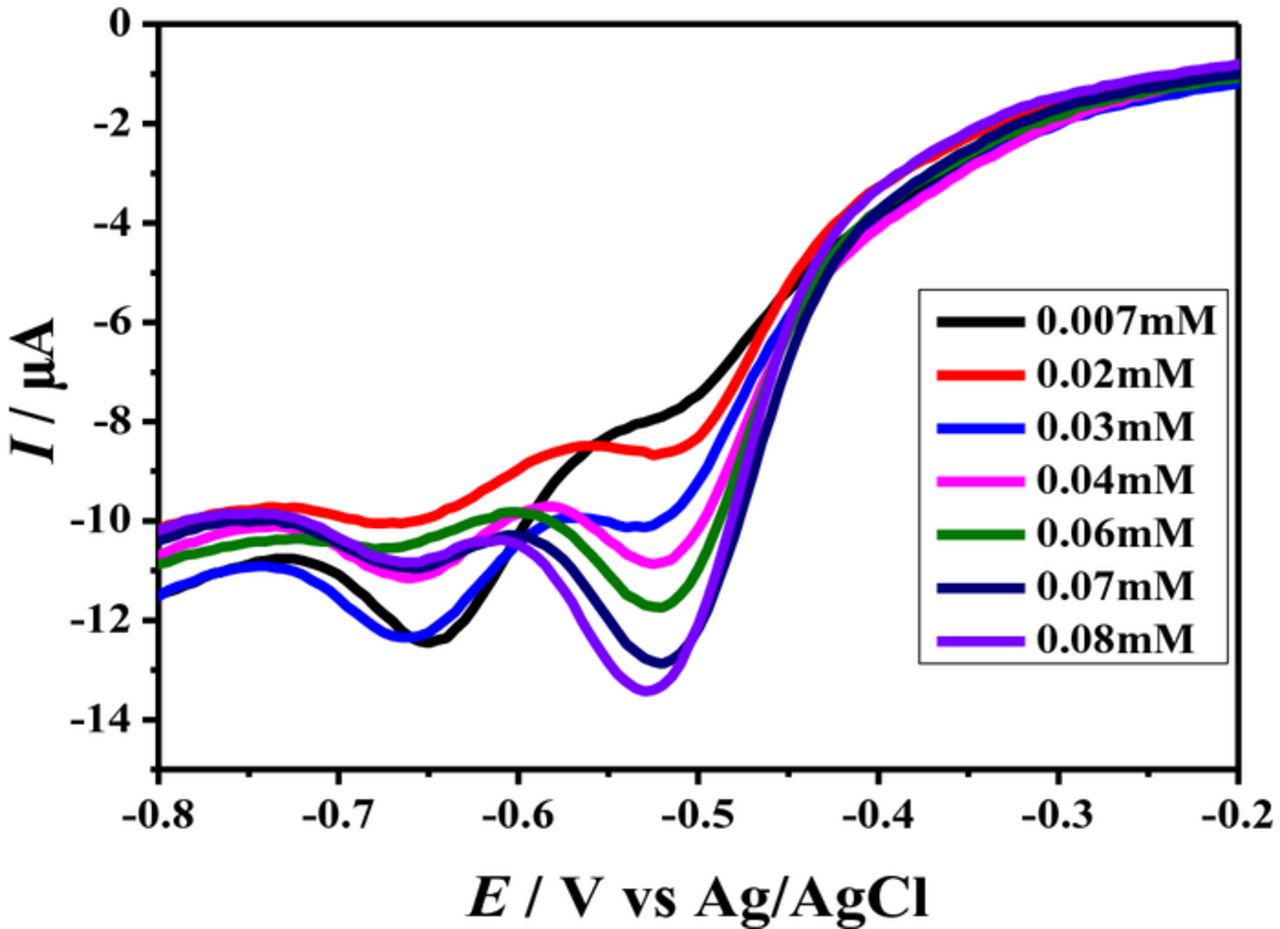
As phenazines are used in pharmaceutical and clinical research so the development of a sensitive method for the detection of their small amount was considered essential. Square wave voltammetry is more sensitive and fast technique than cyclic voltammetry. Moreover, it has the ability of reducing charging current; hence, robust peaks suitable for the detection of very small amount of the analyte can be obtained by using this technique. Based on these considerations square wave voltammetry was employed for the determination of limit of detection (LOD) and limit of quantification (LOQ) of BP and BBP. The reduction signals of compounds were used for examining the concentration effect. Fig. 7 shows decrease of peak current upon dilution of the analyte solution as expected. LOD and LOQ were evaluated using equations;33,38
![Equation ([6])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn6.jpg)
![Equation ([7])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn7.jpg)
where, SD is standard deviation of the intercept and m the slope of current versus concentration plot. LOD and LOQ of BP with values 8.49 μM and 28.32 μM were calculated from the reduction peak around 0.52 V. Similarly, LOD and LOQ with values 6.4 μM and 21.3 μM were evaluated for BBP. These values are in micro molar range like the reported LOD (2.6 μM) and LOQ (8.6 μM) of 1-methoxyphenazine.39 Thus, these analytical parameters in μM range reflect the enhanced sensitivity of the proposed method.
Figure 7. SWVs of different concentration of BP at 100 mVs−1.
Computational studies
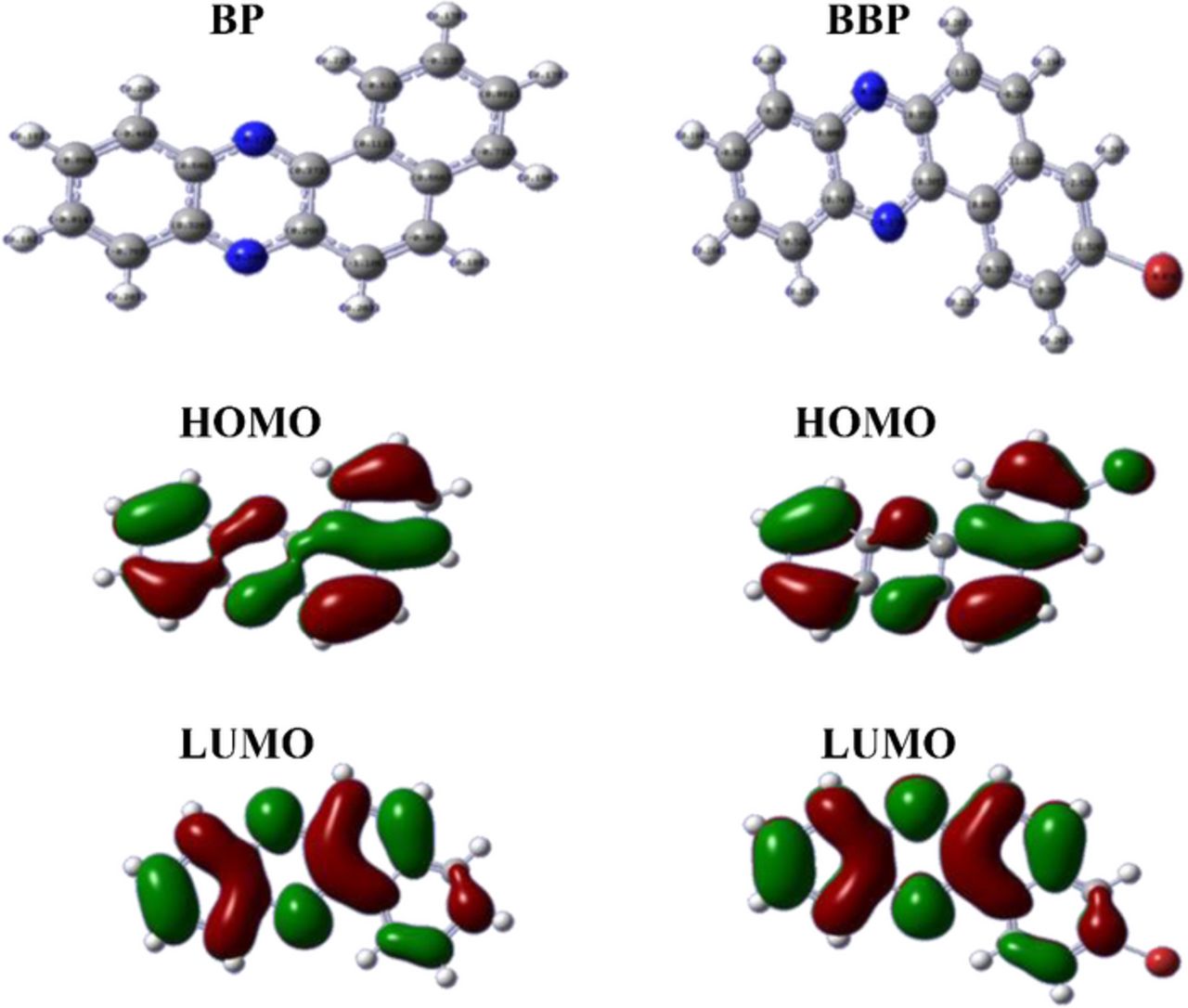
Computational calculations were performed for the identification of favorable oxidation and reduction sites of the compounds and to complement the experimental findings. The geometrical structures of the compounds were first optimized at the hybrid Becke three-parameter Lee–Yang–Parr exchange correlation functional (B3LYP) level with standard 6-31G basis set using Gaussian 3.0 package. Owing to its success in charge calculations, DFT/6-31G was selected for charge calculations. The optimized most stable confirmations were used for charge distribution on atoms of BP and BBP as shown in Fig. 8. The highest negative charge appeared on nitrogen 2 of BP which is indicative of its preferred center for protonation. In contrast, nitrogen 1 of BBP displayed the highest negative charge due to attachment of bromine on benzene ring in the molecule, so DFT signified it as the most favorable atom in the compound for protonation.
Figure 8. Optimized structures of BP and BBP and representation of their HOMO and LUMO.
Computational studies were also performed for the calculation of EHOMO and ELUMO, electronegativity (χ) and global hardness (η). The values of EHOMO and ELUMO listed in Table III indicate the ability of a molecule to donate or accept electrons respectively.40 The ELUMO of BBP and BP are nearly the same; hence, their reduction occurs at almost the same potential under similar solution conditions. Absolute hardness and softness of BBP and BP were calculated to get information about their molecular stability and reactivity. These parameters are related with bandgap as hard molecules have large energy gaps as compared to soft molecules.41 The values indicate that BP and BBP have approximately similar hardness. Absolute electronegativity (χ) and global hardness (η) of the compounds were determined by using equations 8 and 9.42 The ability of a molecule to act as Lewis acid or base can be determined by its χ value. Large χ values characterize a molecule as Lewis acid and vice versa. For any two molecules, electrons are partially transferred from one of lower χ (acting as Lewis base) to that of higher χ.43
![Equation ([8])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn8.jpg)
![Equation ([9])](https://content.cld.iop.org/journals/1945-7111/162/3/H115/revision1/jes_162_3_H115eqn9.jpg)
Dipole moment is used to quantify the polarity of molecules.44 The more polar nature of BBP was confirmed by its higher dipole moment than BP. Total energy calculated by quantum chemical methods gives information about the stability of molecules.45,46 Thus, lower total energy of BP points to its more stability than BBP. Both compounds contained two nitrogen atoms with very much similar chemical environment so it was very difficult to decide the reducible electrophore. Computational studies played a key role in ascertaining the reducible moiety in the compounds. Moreover, the experimental findings were supported and strengthened by computational results.
Table III. Quantum mechanical parameters of BP and BBP.
| EHOMO | ELUMO | ΔE gap | μ | I.E. = | E.A. = | χ | η | |
|---|---|---|---|---|---|---|---|---|
| Compounds | (eV) | (eV) | (eV) | (Debye) | −EHOMO | −ELUMO | (eV) | (eV) |
| BP | −0.233 | −0.097 | 0.135 | 1.985 | 0.233 | 0.097 | 0.165 | 0.067 |
| BBP | −0.240 | −0.104 | 0.136 | 0.663 | 0.240 | 0.104 | 0.172 | 0.068 |
Redox mechanism of BP and BBP
Voltammetric and computational results helped in proposing the redox mechanistic routes of the compounds. The electrochemical reduction of BP was found to occur in a single irreversible step controlled by diffusion. Below pH 8, nitrogen atoms of the molecules having the highest negative charge density are possible to get chemically protonated followed by electrochemical reduction involving the gain of two electrons and one proton as evidenced by half peak width values and slopes of Ep-pH plots. At pH higher than acid base dissociation constant, pKa, the results revealed the reduction of BP to occur by the gain of 2e and 1 H+ resulting in the formation of an electroactive product (see Scheme 1). This reduced product was found to oxidize in a pH dependent manner involving the loss of the same number of electrons and proton as the parent compound. Similarly, the electrochemical oxidation of BP was also investigated and found irreversible. Below pH 8, the compound exists in protonated form and its oxidation leads to the formation of a cation radical which is stabilized by the solvent molecules as shown in Scheme 2. At pH greater than 8, the protonated form of the compound follows the same route of oxidation as mentioned above while the results reveal the original form to oxidize by the loss of two electrons without involvement of protons. The detailed pH dependent redox mechanism of BBP was also proposed and presented in Schemes 3.
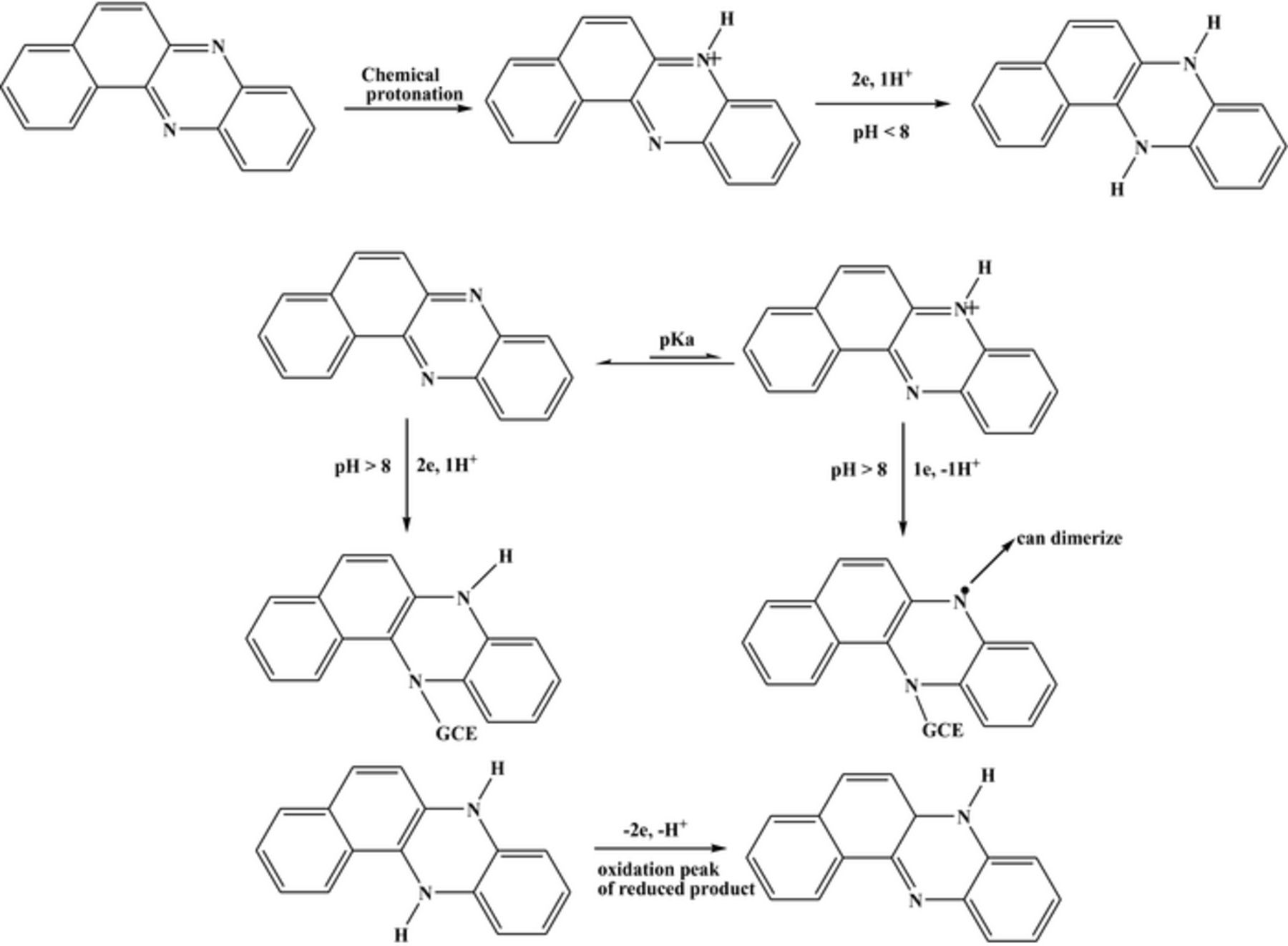
Scheme 1. Proposed reduction mechanism of BP and its reduced product.
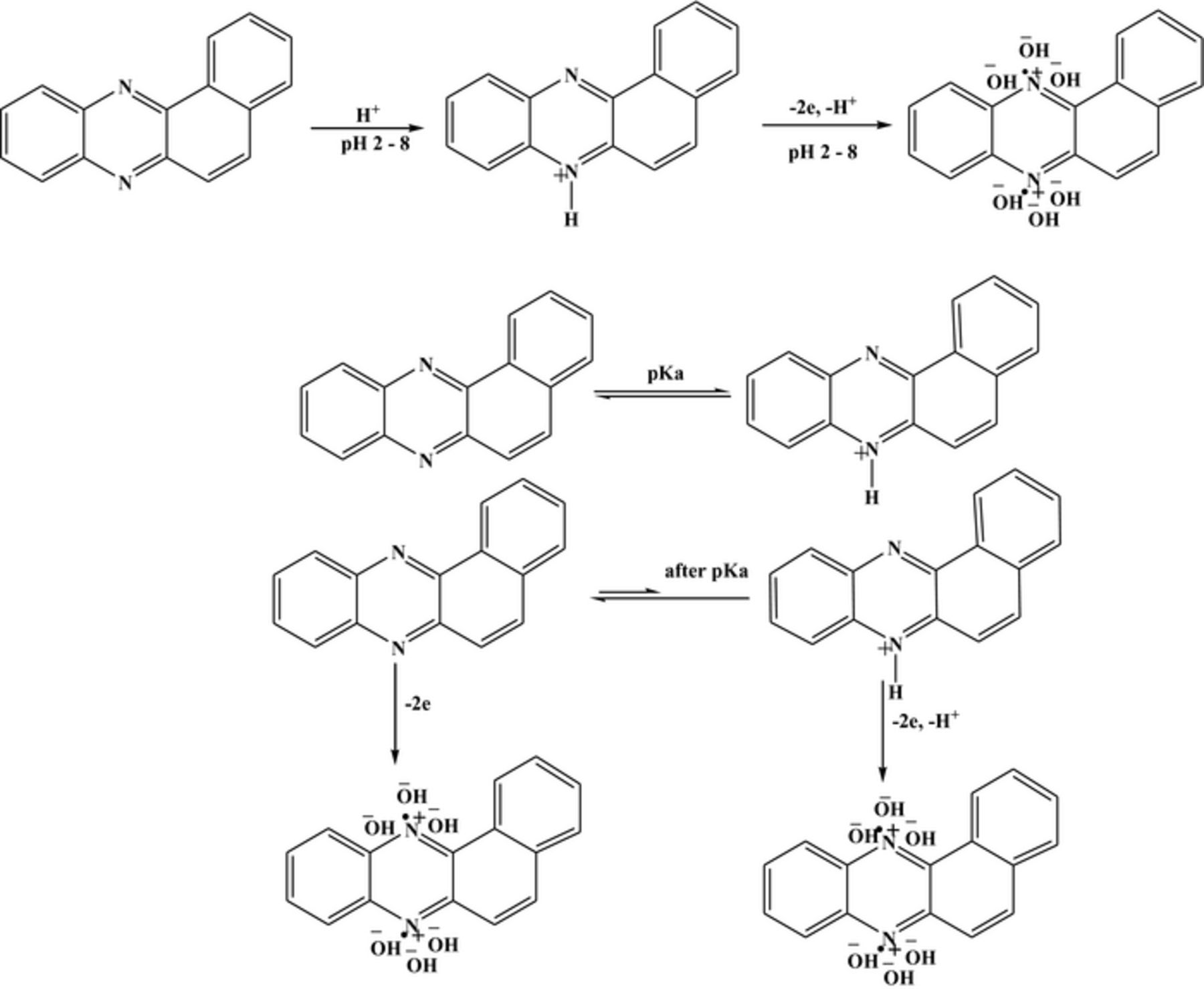
Scheme 2. Proposed oxidation mechanism of BP.
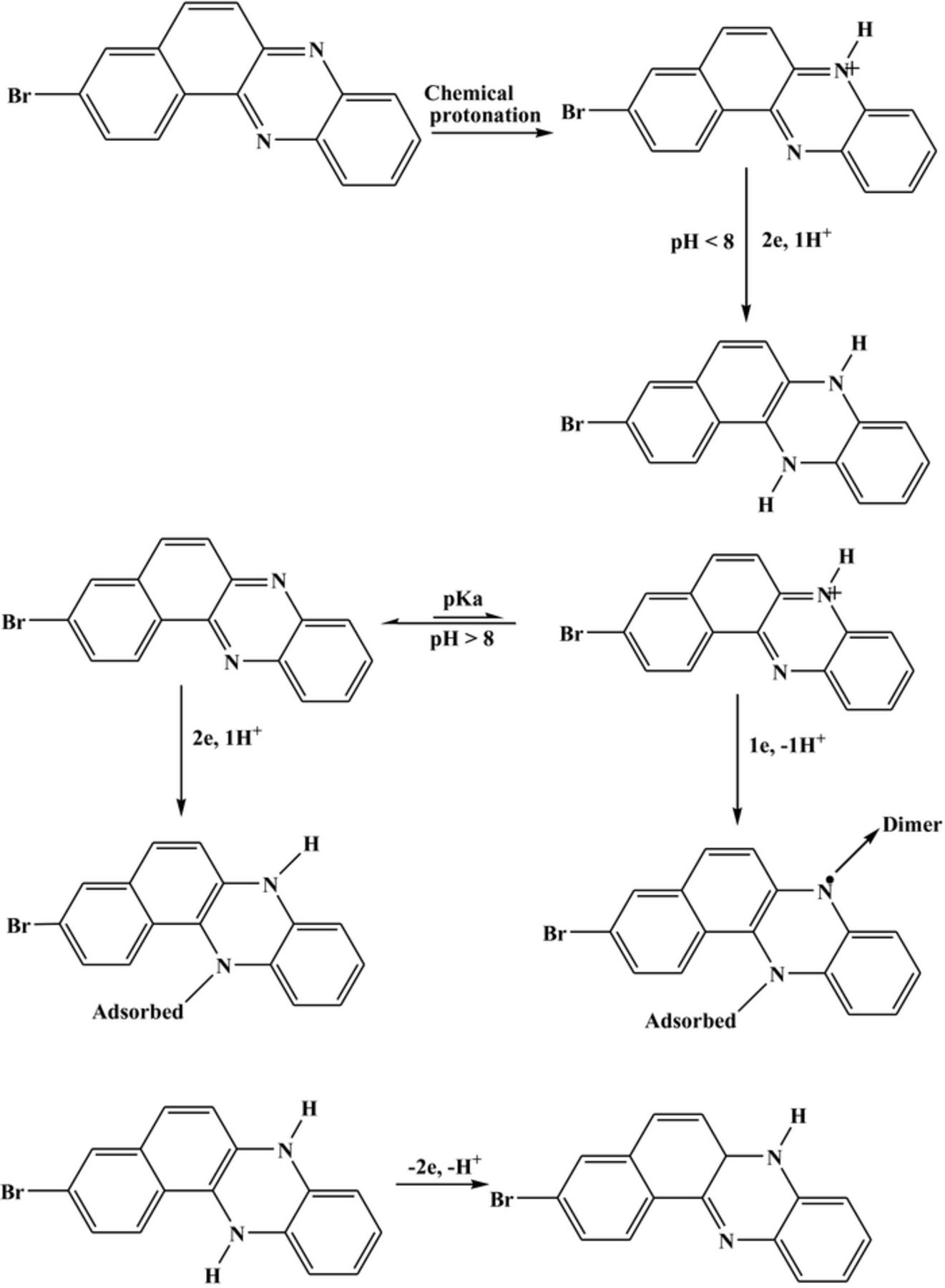
Scheme 3. Proposed redox mechanism of BBP and its reduced product.
Conclusions
Phenazine derivatives were found to reduce in an irreversible, diffusion controlled and pH dependent manner at the surface of GCE. The irreversible nature of the reduction processes was confirmed from shift in reduction potentials with increasing scan rate, value of electron transfer rate constant, (Epc – Epa)/n greater than 212 mV and Ipc/Ipa ≠ 1. Likewise, the results revealed irreversible and pH dependent oxidation of the reduction products of BP and BBP. Various useful kinetic and thermodynamic parameters were evaluated from the cyclic voltammograms obtained at different temperature values. Positive values of Gibbs free energy and enthalpy changes revealed the non-spontaneous and endergonic nature of redox processes. The positive value of entropy change revealed the entropy driven reduction reaction of BP. The computational studies reveal that BP is more polar so its greater solvation makes it more ordered than the product, hence, results in positive ΔS in contrast to BBP. Computational investigations helped in identification of the most favorable reduction sites of the compounds. The lower oxidation potentials and consequent facile oxidation of the selected phenazines in media of higher pH predict thermodynamic feasibility of their electron transfer reactions in biological systems. On the basis of experimental and theoretical findings, redox mechanism of the selected phenazines were proposed which are expected to provide useful insights about the hidden pathways by which this class of compounds play its biochemical role in living organisms.
Acknowledgments
The authors gratefully acknowledge the financial support of Higher Education Commission of Pakistan through project number 20-3070, Quaid-i-Azam University and the University of Toronto Scarborough, NSERC. The authors would like to extend their sincere appreciation to the Deanship of Scientific Research at King Saud University for funding this Research through the group project No. RGP-VPP-345.