Abstract
A witch ball is a reflecting sphere of glass. Looking into the disk that it subtends, the whole sky can be seen at one glance. This feature can be exploited to see and photograph the squint Moon illusion, in which the direction normal to the illuminated face of the Moon—its 'attitude vector'—does not appear to point towards the Sun. The images of the Sun and Moon in the disk, the geodesic connecting them, the Moon's attitude, and the squint angle (distinct from the tilt), can be calculated and simulated, for all celestial configurations and viewing inclinations. The Moon direction antipodal to the Sun, corresponding to full Moon, is a singularity of the attitude vector field, with index +1. The main features of the witch ball images also occur in other ways of imaging the squint Moon.
Export citation and abstract BibTeX RIS

Content from this work may be used under the terms of the Creative Commons Attribution 3.0 licence. Any further distribution of this work must maintain attribution to the author(s) and the title of the work, journal citation and DOI.
1. Introduction
Reflecting glass spheres have interesting optical properties [1]. And they have applications: hung in the windows of country houses to repel an approaching witch (who fears her image); and in country gardens, to reflect the whole sky, enabling delicate clouds and sky colours to be seen more clearly [2] (because minification increases contrast gradients). Looking into such a 'witch ball' or 'garden globe', we see a disk of light, reflected from the entire 4π sphere of directions: forwards near the edge, and, in the center, the head or camera containing the observing lens obscuring light from behind (figure 1).
Figure 1. Images in a witch ball; (a) viewed horizontally, (b) viewed vertically. (These pictures have been reversed left-to-right, to reverse the mirror reversal.)
Download figure:
Standard image High-resolution imageHere I describe another use for such a 'witch ball' or 'garden globe': to study the squint Moon [2–4] illusion. We know that the Sun illuminates the Moon, and, depending on the Moon's phase (crescent, half, gibbous), we see part of the lit hemisphere, corresponding to the relative positions of the Sun and Moon in the sky. Therefore we expect the lit side of the Moon to point towards the Sun. But it does not: usually, it points above the Sun. This is the squint (figure 2). It is commonly seen when the Sun and Moon are in the sky at the same time; but it is also particularly clear for the gibbous Moon after sunset: we know that the Sun is below the horizon, yet the Moon sometimes points upwards.
Figure 2. (a) Schematic view of the sky illustrating the squint Moon, with the projection from the direction sphere deliberately unspecified. (b) Photograph (perspective projection) showing the Moon just after first quarter, when the Sun (far out of shot) is setting; the inset shows the Moon magnified; it points upwards, squinting above the Sun.
Download figure:
Standard image High-resolution imageIt is obvious that the squint is an illusion: in three-dimensional space the normal to the lit face must intersect the Sun, because light travels in straight lines. One well-known [2] way to confirm this, and thus dispel the illusion, is to hold a string taut with one end at the Moon and the other at the Sun: at the Moon, its direction coincides precisely with that of the lit face.
Several important aspects of the illusion have been studied in detail: its dependence on perception as well as cognition [5]; the need to move one's head in order to follow the direction from the Moon to the Sun when they are too far apart in the sky to be seen simultaneously [6–8]; the extent to which the illusion depends on standing upright and looking horizontally, and whether the illusion requires a straight horizon [9]; and perspective and orthogonal projections associated with vision and photography [6, 7, 10].
My emphasis here is different: to understand how the squint appears when viewed in a witch ball, and thereby illustrate its persistence in a variety of circumstances: it is robust. Imaging the sky in this way has several advantages: the Sun and Moon can be seen simultaneously in the globe's disk, without the need to move one's head. By changing the direction in which the globe is viewed, the horizon can appear straight or curved, enabling exploration of different ways in which the squint appears. And, as explained in section 2, the optics is relatively simple, enabling an unambiguous definition and calculation of the squint, and simulation of how it appears in the witch ball, in section 3.
Why does the squint appear at all? The Sun is much more distant than the Moon, and this affects the shape of the illuminated Moon: its phase. But both Sun and the Moon are so far from us that we do not directly perceive their distances in three-dimensional space, but rather their directions in the sky, relative to the Earth. Directions can be represented as unit vectors on the celestial sphere, that is, points on the Earth-centered sphere of directions (figure 3). On this sphere, the straight line from the Moon to the Sun in three-dimensional space is represented by a great circle: a geodesic. We do not perceive the sphere directly. Rather we see its projection onto a skyview: some perceptual space, or a space defined by an imaging system—for example a panoramic picture created by stitching together photographs taken in different directions, or the reflected image in the witch ball, to be studied here, where the skyview is a disk.
Figure 3. Plane containing the Moon, Sun and Earth, and its intersection with the unit sphere of directions.
Download figure:
Standard image High-resolution imageEvery projection from the sphere to some skyview, e.g. a plane, introduces distortion. Usually the geodesic great circle will be projected as a curve. The direction in which the Moon appears to point is the tangent to the geodesic at the location of the Moon. For this direction, which can alternatively be described as the 'symmetry axis of the illuminated face', or 'the normal to the lit face' or 'the perpendicular bisector of the line joining the horns of the Moon', I introduce, as a less cumbersome term, the Moon's attitude (it is also called the 'Moon pointer' [7]); see figure 1. For curved geodesics, the attitude does not point to the expected position of the Sun—meaning that it does not point along the straight line connecting the Moon to the Sun in the skyview. The squint is the discordance between these two directions, and its magnitude can be represented as the angle between them. (For perspective and orthogonal projections [7], straight lines in space project to straight lines in the plane, so the geodesics are not curved—but angles are distorted, so the squint still appears, albeit described differently.)
The squint angle depends on the projection, that is, on our perceptual space (which need not be the same for everybody or at all times), and on the imaging system used. But the squint phenomenon occurs for every projection; one example, different from the witch ball image to be studied here, is zenith-centered sterographic projection [4], roughly modeling the squint seen lying down and looking up. And as we will see, the squint can be positive, negative, or zero (when the axis of projection intersects the geodesic). This variability contrasts with the tilt, namely the angle between the attitude and the horizontal, which can be calculated directly on the direction sphere (section 3), regardless of how it is imaged. (To avoid a potential source of confusion, I note that the phenomenon here called the squint Moon, following [3], is often called the tilted Moon.)
The squint seen in a witch ball can be captured photographically, as described and illustrated in section 4. When the Moon is close to full, i.e. when the Sun and Moon are opposite in the sky, the attitude is very sensitive to the phase. This is the antipodal singularity, explained in section 5. Some subtle details of the images in a witch ball are explained in the appendix.
2. Witch ball imaging
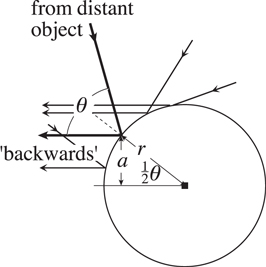
Looking into the globe, which has radius r, we see objects reflected in a disk, also of radius r. Referring to figure 4, consider a ray reflected after arriving from a direction θ. It suffices to restrict the analysis to a distant viewer, so that reflected rays are always in the same direction ('backwards'); for a viewer at a finite distance [1], the calculations are a little more complicated, but all essential features, including the simulations to follow in section 3, are the same.
Figure 4. Geometry of reflection in a witch ball.
Download figure:
Standard image High-resolution imageIt is obvious from the law of specular reflection and figure 4 that the distance a of the location of the image on the observation disk is [2]
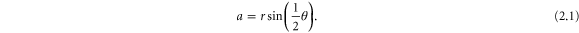
(It is worth noting that the stereographic projection outlined elsewhere [4] corresponds to replacing 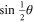 by
by 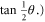 More generally, the image in the disk, of light arriving from a distant object in a direction
More generally, the image in the disk, of light arriving from a distant object in a direction
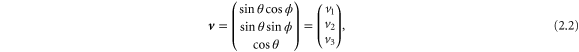
where the origin of polar coordinates is backwards, is
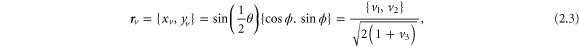
in which the x axis runs East–West and the y axis North–South. Here and hereafter we will usually take r = 1, with no essential loss of generality.
Let the polar angles (π/2–elevation) of the Moon and Sun in the sky be θm and θs, with corresponding azimuths ϕm and ϕs. This corresponds to spherical polar coordinates with axis vertically upwards. The corresponding direction vectors of the Moon and Sun are
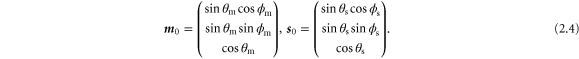
With a view inclined at an angle α to horizontal (where α > 0 is looking downwards, so α = π/2 corresponds to viewing the overhead sky in the disk), the transformed directions of the Moon and Sun required for imaging in the witch ball are
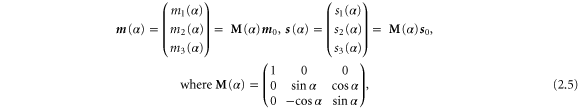
corresponding to a rotation α about the x axis. Henceforth we will not always indicate the α dependence explicitly.
On the disk, the positions of the Moon and Sun are, according to (2.3),
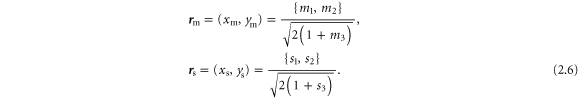
Similarly, the horizon appears on the disk as a curve given parametrically by
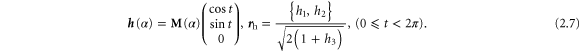
So, looking down (α = π/2), the horizon is a circle of radius r/√2, and looking horizontally it is the segment |x| ≤ 1, of the x axis y = 0.
As is easily shown [1], the projection corresponding to (2.1) is area-preserving: a given solid angle dΩ in the sky maps to an area dA on the disk, such that dA/dΩ = r2/4, independent of position in the sky or on the disk. In cartography, this is Lambert's azimuthal equal-area projection of 1772.
3. Simulating the squint
On the direction sphere (figure 5), the geodesic connecting the Moon to the Sun is the curve
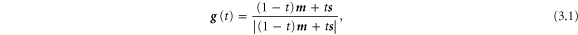
where the parameter value t = 0 corresponds to the Moon and t = 1 to the Sun. On the disk, the corresponding curve is
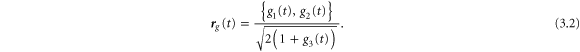
Figure 5. Geometry on the direction sphere: Moon, Sun, geodesic, attitude, and tilt τ.
Download figure:
Standard image High-resolution imageThe attitude vector A is the direction of the geodesic (figure 5) at the location of the Moon. A short calculation gives this vector in the witch ball skyview as
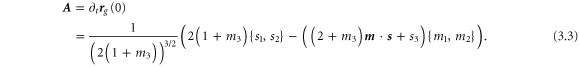
The squint is the angle σ between the vector A and the vector rs–rm connecting the Sun and Moon in the skyview, namely
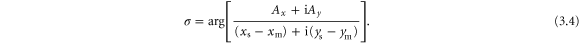
(This way of expressing the angle eliminates the ambiguities of trigonometric functions.)
The squint angle σ is a function of the five angles θm, ϕm, θs, ϕs, and α. Figure 6 shows the dependence on the Moon's azimuth ϕm, and viewing elevation α, for Sun elevation 10° (θs = 80°) and in the West (ϕs = 0), and Moon elevation 45° (θm = 45°). The squint is usually positive, but is negative for some higher viewing elevations; this will be explained later.
Figure 6. Squint angle σ as a function of the Moon azimuth ϕm and viewing elevation α, for Moon elevation 45°, and Sun elevation 10°and azimuth 0. The intersection with the plane σ = 0 indicates the line across which σ changes sign.
Download figure:
Standard image High-resolution imageTo simulate the phase of the Moon, we note that the circular boundary of the illuminated hemisphere appears from Earth (figure 3) obliquely, as an ellipse with axis ratio 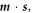 and we see the part that faces us—the terminator line—as a half-ellipse. Therefore the Moon's boundary shape can be simulated as the union of this half-ellipse and a semicircle.
and we see the part that faces us—the terminator line—as a half-ellipse. Therefore the Moon's boundary shape can be simulated as the union of this half-ellipse and a semicircle.
Figure 7 shows some simulations of the Sun and Moon as seen in the witch ball, for nine configurations corresponding to the squint angle calculated in figure 6, with the Sun position and the Moon elevation fixed, and the crescent, half and gibbous Moon (different azimuths ϕm), for three different viewing angles. The top row shows the sky looking down, i.e. seeing, reflected in the witch ball, what would appear without the witch ball when lying down and looking up. The middle row shows the same configurations viewed obliquely. The bottom row shows the horizontal view.
Figure 7. Simulations of the sky seen in a witch ball. Red arrows: the Moon's attitude; dashed curves: the geodesic from the Moon to the Sun; black dots: the zenith; white curves: the horizon; open circles: the viewing axis. In (a), (b) and (c), the viewing elevation is α = 90°; in (d), (e) and (f), α = 45°; in (g), (h) and (i), α = 0. The Sun elevation is 10° (θs = 80°), and its azimuth is ϕm = 0; the Moon elevation is 45°(θm = 45°); in (a), (d) and (g) the Moon azimuth is ϕm = 50°; in (b), (e) and (h) ϕm = 90°; in (c), (f) and (i) ϕm = 130°.
Download figure:
Standard image High-resolution imageFor all simulations except figure 7(e), the squint is clearly indicated by the curvature of the geodesic: the Moon's attitude (red arrows) does not point to the Sun. Figure 7(e) marks the change of sign of the squint angle σ from positive (Moon points above the Sun) in figures 7(f)–(i) to negative in figures 7(a)–(d). Zero squint corresponds to the viewing axis lying on the geodesic; I owe this insight (which also holds for stereographic and other projections) to Professor Vager (private communication). The transition is illustrated in figure 8, by a fixed Moon and Sun configuration viewed from three directions α.
Figure 8. As figure 7, illustrating the sign change of squint σ as the viewing axis crosses the geodesic, for Moon elevation 40° (θm = 50°) and azimuth ϕm = 160°, with the Sun on the horizon (θs = 90°) and azimuth ϕs = 45°, for viewing elevations (a): α = 10° (σ > 0); (b): α = 33.212° (σ = 0); (c): α = 60° (σ < 0).
Download figure:
Standard image High-resolution imageThe squint angle σ, quantifying the discordance between the Moon's attitude A and the direction from the Moon to the Sun in the skyview, is different from the tilt τ, which is the angle between A and the horizontal. We can calculate τ using the direction sphere. From figure 5, it is clear that
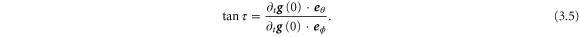
From (3.1), the derivative is
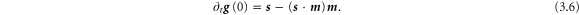
This gives, after a short calculation based on (2.4) and (2.5),
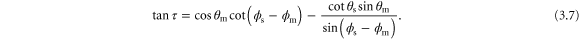
4. Observations
As already mentioned, there are at least two advantages of using a witch ball to see the squint. Even when the Sun and Moon are far apart in the sky they can be seen reflected in the ball without needing to move one's head. And the projection onto the viewing disk is a consequence of elementary optics and does not require assumptions about the skyview that corresponds to our visual perception.
But there is a disadvantage: the Moon occupies a tiny fraction of the sky, namely
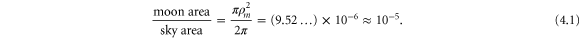
Therefore it appears in the globe as a tiny dot, which must be magnified in order to discern the phase and therefore the attitude. This difficulty is shared with all ways of photographing the squint, as published pictures illustrate, for example in [5, 11, 12] (the conventional photograph in figure 2(b) shows the Moon but not the Sun, which is on the horizon, far out of shot). However, this difficulty can be overcome, and the Moon's attitude identified, as figure 9 illustrates.
Figure 9. The sky, photographed in a witch ball just before sunset in Bristol on 28 December 2014 (a clear day slightly before half-Moon). In (a) and (c), the location of the Moon is indicated by a red circle. In the magnified pictures (b) and (d), the insets show further magnifications indicating the attitude A (red arrow); the squint is obvious from the red line continuing A and the geodesic sketched as the yellow dashed curve: σ is positive in (a) and (b), and negative in the higher-inclination view (c) and (d). (These pictures have been reversed left-to-right, to reverse the mirror reversal.)
Download figure:
Standard image High-resolution image5. The antipodal singularity.
The attitude A is defined in terms of the orientation in the sky of the lit face of the Moon. At the moment of full Moon, when the Sun and Moon are in opposite directions relative to the Earth, that is, antipodal on the direction sphere, the lit face points towards us. Therefore its orientation in the sky (on the direction sphere or in any skyview) is undefined. This corresponds to a singularity of the squint, because as the Moon passes full its gibbous face reverses, and so does the vector A. Indeed, for configurations close to full, A can have any orientation.
The antipodal singularity is illustrated in figure 10(a). This shows several positions of the gibbous Moon, viewed obliquely in the witch ball after sunset. It is clear that A rotates through 360° around the direction antipodal to the Sun. This means that on the direction sphere, or any projection of it such as the witch ball projection, the vector field A has a singularity of index +1 at the antipode. This is also a singularity of the squint angle on the witch ball, because σ also changes by 360° in a circuit of the antipode.
Figure 10. (a) Several configurations of the gibbous Moon, showing the attitude vectors (red arrows) surrounding the full Moon (white disk without an arrow), antipodal to the fixed position of the Sun after sunset (60° below the horizon, i.e. θs = 150°, with azimuth ϕs = 45°), viewed in the witch ball at elevation α = 30°; the horizon is the white curve. (b) As (a), showing the attitude vector field A plotted for 1000 random Moon directions θm, ϕm, showing index +1 singularities at the Sun and its antipode.
Download figure:
Standard image High-resolution imageFigure 10(b) shows the attitude field A for fixed Sun position after sunset, plotted for random Moon directions above and below the horizon. The antipodal singularity is obvious. Also obvious is a singularity at the direction of the Sun, also of index +1, consistent with the Poincaré–Hopf theorem [13] that any vector field on a sphere must have singularities whose total index is +2. But this singularity of the field A is not a singularity of the squint, because close to the Sun (i.e. near new Moon) the Moon–Sun direction is almost parallel to A, so the squint is small for a thin crescent Moon, and vanishes at new Moon.
6. Concluding remarks
In one sense, choosing the witch ball to observe and record the squint is a jeu d'esprit, a celebration of the International Year of Light, further exploring one of several phenomena treated briefly elsewhere [4]. In light of the fact that the squint, as seen by eye standing upright looking directly at the Moon, has been explained by other authors [5–7, 10], this choice might seem superfluous, even eccentric. But there are several reasons for it. When concentrating on the simplest way in which the squint appears, it is easy not to appreciate that it is a more general phenomenon. Other ways of perceiving the squint reveal that several features, which some authors have thought essential in explaining it, are in fact not: being upright, perceiving the horizon as straight, using perspective projections to model the simplest photography, moving one's head to shift one's gaze from the Moon to the Sun.
This shift of emphasis, to the wider class of squints, leads to the geometric way of understanding the phenomenon, based on the general class of projections from the direction sphere (or the straight line of light from the Sun to the Moon in three-dimensional space), in which geodesics appear as different curves. Further, it leads to the identification of the antipodal singularity as a central aspect of the squint. The witch ball is a device that exemplifies this generality: although the optical projection is area-preserving, it is neither conformal (preserving local angles) nor perspective or orthogonal (preserving straight lines); it allows for different viewing orientations, in almost all of which the horizon is curved; it does not require eye or head movement; and it does not require the choice of a stitching algorithm to create a panoramic photograph. Yet the squint persists, and can be observed and recorded.
Acknowledgments
I thank O Abramson, A Myers, A K Myers-Beaghton, B Rogers and Z Vager for introducing me to the squint and for helpful (and occasionally robust) correspondences. The early stages of this work were supported by an Emeritus Fellowship from the Leverhulme Trust.
Appendix: Witch ball caustic
To study the image of a distant point, consider all the rays incident from a distant object and emerging in different directions θ after being reflected. The image is virtual, that is, inside the witch ball. From figure 4 (with the arrows reversed), the equation of the virtual ray that emerges at θ is (again with r = 1)

These rays do not intersect at a focal point. Instead, they touch a caustic surface, obtained by rotating a caustic line about its symmetry axis. The caustic is the envelope defined by
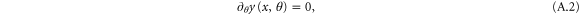
together with (A.1). A short calculation gives its equation parametrically as
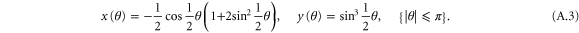
This is a cusped curve, shown in red in figure 11, which has been turned to correspond to the family of rays for which θ is the direction of incidence.
Figure 11. Virtual caustic (red curve), for a family of rays from a distant source, formed by the continuation of all the reflected rays inside the ball. The blue dotted curve is the segment of the caustic that enters the eye or camera lens.
Download figure:
Standard image High-resolution imageTherefore the ball images each point in the sky onto a curve (or, when rotated, a 'spun cusp' surface). Nevertheless, we see sharp images—and not only in the witch ball but also in most curved surfaces, for example distorting fairground mirrors. The reason is explained elsewhere [1] and simply outlined here. The emerging rays forming the witch ball image come not from the whole caustic but from the segment (dotted blue in figure 11(b)) whose tangent rays enter the eye pupil or the camera lens. The projection of this segment normal to the viewing direction is the geometrically blurred image, but usually falls below the Rayleigh resolution limit and so cannot be resolved. The image of the sky, namely the union of location of the effectively point images for each value of θ, lies on a surface whose distance from the plane normal to the optic axis and including the center of the ball, varies from r/2 (for θ = 0) to zero (for θ = π). The distance of these images from the axis is given by (2.1).