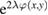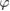Abstract
The aim of this article is to investigate the uniqueness of solution of an inverse source problem for ultrahyperbolic equations. We first reduce the inverse problem to a Cauchy problem for an integro-differential equation and then by using a pointwise Carleman type inequality we prove the uniqueness.
Export citation and abstract BibTeX RIS
1. Introduction and the main result
In this article, we consider an inverse problem for an ultrahyperbolic equation. One of our motivations to deal with this equation is its interesting structure from the point of view of the theory of partial differential equations. For instance, depending on the specific form of initial conditions, solutions possess both hyperbolic and elliptic properties (see [15]). Another motivation is recent discussions on the possibility of physics in multiple time dimensions, (e.g. [4, 20, 21]). Namely, in some superstring theories which attempt to unify the general theory of relativity and the quantum mechanics, extra dimensions are required for the consistency of theory. When the presence of more than one temporal dimension is considered, the mathematical model occurs as an ultrahyperbolic equation (e.g. [9]). More precisely, the paper [9] asserts that the equation in a form of

is of central physical importance, which describes the dynamical evolution of many physical quantities of classical and quantum field theories including the components of the electromagnetic fields in the case of a single time dimension, while the equation in a form of
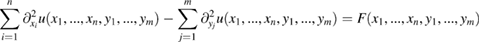
is fundamental where 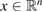 and
and 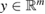 are, respectively, space-like and time-like variables.
are, respectively, space-like and time-like variables.
Throughout the paper, we set
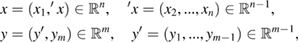
and use the following notation
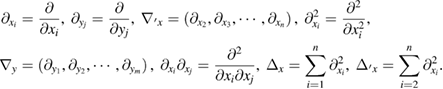
Moreover for short, we write
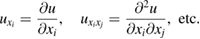
Henceforth for 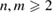 , let
, let  be a bounded doman and
be a bounded doman and  where I is an open bounded interval and
where I is an open bounded interval and 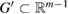 is a bounded domain. We set
is a bounded domain. We set
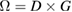
and we assume that 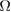 is supported by the plane x1 = 0, that is, for any
is supported by the plane x1 = 0, that is, for any  there exists a cone which is contained in
there exists a cone which is contained in 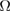 and includes the point
and includes the point  , and whose base lies on the plane x1 = 0.
, and whose base lies on the plane x1 = 0.
Similarly to [9, 20, 21], we here consider an ultrahyperbolic equation in  , which is associated with general geometry in the time variables y :
, which is associated with general geometry in the time variables y :
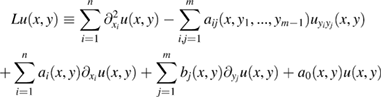

in the domain 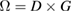 .
.
Throughout we assume that we can choose constant M > 0 such that

and  ,
,  and
and 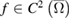 . We note that in this article, we do not assume the ellipticity for
. We note that in this article, we do not assume the ellipticity for 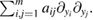
The purpose of this article is to investigate the uniqueness of solution of the following problem:
Inverse source problem.
For given 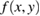 and
and  , find a pair of functions
, find a pair of functions  in
in 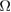 satisfying equation (1), Cauchy data
satisfying equation (1), Cauchy data
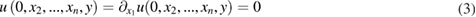
and the additional information
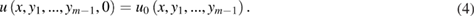
This is an inverse problem of determining a factor g which is independent of one component y m of the time-like variable of the right-hand side of (1). When we admit the multiple time dimensions related to a superstring theory (e.g. [9, 20, 21]), as a governing equation, we introduce an ultrahyperbolic equation (1) and then we should discuss an original cause initiating dynamical evolution of physical quantities. In our case, the right-hand side  in (1) is assumed to be such a cause and our inverse problem is the determination of a factor g.
in (1) is assumed to be such a cause and our inverse problem is the determination of a factor g.
Our main result is stated in theorem 1 and asserts that our data can determine the factor g uniquely.
Theorem 1. We assume that  and there exists a constant
and there exists a constant 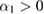 such that
such that
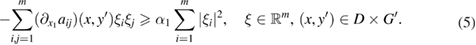
Then the inverse source problem has at most one solution  such that
such that 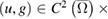
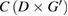 .
.
For the uniqueness in the inverse problem, we need to assume (5) and in general we cannot expect the uniqueness for aij satisfying ellipticity condition and (2) even for the case m = n = 1. We can interpret condition (5) in the case of m = 1 that wave propagation speed increases at a neighborhood of the plane x1 = 0, which implies the absence of waveguides in a medium with x1 > 0 and as a related work, see Amirov and Yamamoto [3].
Inverse problems for ultrahyperbolic equations were studied in [1, 2, 6, 11], where the key method is based on weighted a priori estimates and was firstly developed by Bukhgeim and Klibanov [6]. A uniqueness theorem for ultrahyperbolic equations is given by [6] for a bounded domain with Dirichlet and Neumann type condition on a part of the boundary. In [1] and [2], uniqueness is investigated in an unbounded domain with an additional information for the solution of direct problem at y = 0. In [11], Hölder stability estimates were obtained in a bounded domain by some lateral boundary data. A major difference of our work from the existing results is that, in the inverse problem, additional information is given only at y m = 0.
As for the direct problem (1) and (3) with given fg, it is known that the problem of determination of the function u from relations (1) and (3) is ill-posed in the Hadamard sense (see [16], chapter IV). By using the mean-value theorem of Asgeirsson (see e.g. [13]), it was shown by [8] that the existence of solutions fails if the initial conditions are not properly prescribed. We refer to [7, 10, 17–19], as for the uniqueness results for various Cauchy, Dirichlet and Neumann problems for ultrahyperbolic equations. Finally, in [9] it is proved that under a nonlocal constraint, the initial value problem is well-posed for initial data given on a codimension-one hypersurface.
This paper consists of four sections and one appendix. In section 2, we state proposition 1 which is the key Carleman estimate for the proof of theorem 1 and the proof of the proposition is given in section 4. In section 3, we prove theorem 1 on the basis of proposition 1. In appendix, we prove two lemmata which are used for the proofs of proposition 1 and theorem 1.
2. Key Carleman estimate
In order to prove theorem 1, the key tool is an Carleman type inequality which will be presented in proposition 1 below.
We now introduce a Carleman weight function in the form of 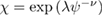 where
where
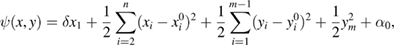
where 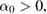
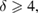
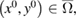 and
and 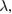
 are large positive parameters.
are large positive parameters.
We define the domain 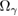 as a level set by
as a level set by 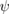 . More precisely, we set
. More precisely, we set
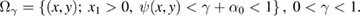
Then we see
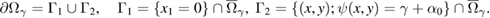
First, we reduce equation (1) to a more suitable form by introducing a new variable  , that is,
, that is,  , where
, where 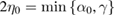 . Without loss of generality, we assume that
. Without loss of generality, we assume that  i.e.
i.e. 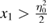 , and so we have
, and so we have 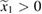 . Then, for the new function
. Then, for the new function 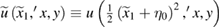 , by using the relations
, by using the relations
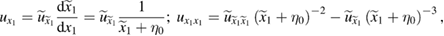
we have
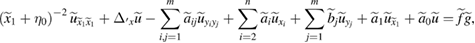
where 
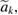
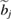 ,
, 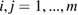 ,
,  are functions in
are functions in 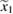 ,
, 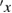 and
and 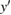 or y , and given by
or y , and given by 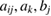 ; for example,
; for example, 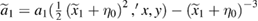 , and
, and 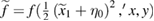 and
and 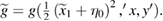
For the sake of simplicity, let us denote 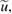
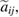
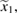
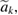
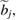
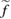 ,
,  by
by  aij, x1, ak, bj ,
aij, x1, ak, bj ,  g respectively, where
g respectively, where 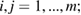
 Then we can write
Then we can write
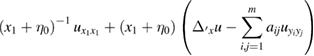
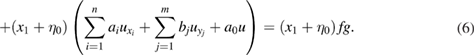
We set
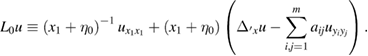
Henceforth C > 0 denotes generic constants which are independent of 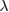 and
and 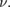
Then we can have the following, which is the key for the proof of theorem 1.
Proposition 1. (Carleman estimate for an ultrahyperbolic equation) Let condition (5) be satisfied and 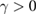 be so small that
be so small that
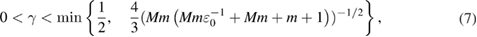
where 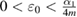 . Then there exists a constant
. Then there exists a constant  such that for any
such that for any 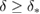 and
and 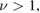 there exists a positive number
there exists a positive number 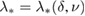 such that for any
such that for any 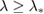 , the following estimate holds
, the following estimate holds
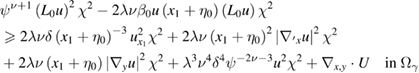
for all  . Here
. Here  and the vector-valued function U satisfies the following inequality:
and the vector-valued function U satisfies the following inequality:
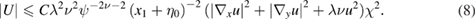
The Carleman estimate proposition 1 is an estimate which is uniform in large parameter  , that is, the constant C > 0 is independent of
, that is, the constant C > 0 is independent of 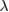 provided that
provided that 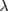 is sufficiently large. This uniformity is essential for the application to the inverse problem.
is sufficiently large. This uniformity is essential for the application to the inverse problem.
As for Carleman estimates for ultrahyperbolic equation (1) with constant principal parts aii = 1 and aij = 0 for  , see section 2 of chapter 4 of [1, 2] and section 4 of chapter IV of [16]. As for a Carleman estimate for an ultrahyperbolic equation with variable aij, see also Romanov [19] which assumes the ellipticity for aij unlike our paper.
, see section 2 of chapter 4 of [1, 2] and section 4 of chapter IV of [16]. As for a Carleman estimate for an ultrahyperbolic equation with variable aij, see also Romanov [19] which assumes the ellipticity for aij unlike our paper.
We note that reflecting a similar property to the hyperbolicity, the ultrahyperbolic equations require some condition (5) to the principal coefficients aij for proving a Carleman estimate.
As general but very limited references on Carleman estimates, we refer, for example, to Bellassoued and Yamamoto [5], Hörmander [12], and Klibanov and Timonov [14]. Our Carleman estimates are different from more common Carleman estimates in e.g. [5, 12] in the following senses:
- Our Carleman estimate asserts a pointwise inequality, while the conventional Carleman estimates are proved in terms of weighted L2 -integrals of solutions u and boundary data.
- In conventional Carleman estimates, the weight function has a form
 with suitable
with suitable  , and the estimate holds uniformly in all large
, and the estimate holds uniformly in all large  .
.
One can prove a conventional type of Carleman estimate for (1), and we can apply both types similarly to inverse problems and establish the uniqueness for the inverse problem. We note that our weight function is the same as in [1, 2], while [16] applies a weight in the form of 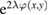 .
.
3. The proof of theorem 1
Once that a relevant Carleman estimate proposition 1 is established, we can apply it for the proof of the uniqueness of the inverse problem by the method originating from Bukhgeim and Klibanov [6]. As for such a method, see also [3, 5, 14]. In this section, postponing the proof of proposition 1 to section 4, we first complete the proof of theorem 1.
Let 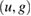 be a solution to (1), (3) and (4) with
be a solution to (1), (3) and (4) with  in
in  . Since
. Since 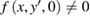 and
and 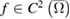 , there exists a number
, there exists a number 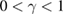 such that
such that  also in
also in 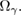 We assume that
We assume that  , which was introduced before, satisfies this condition. We define a new unknown function
, which was introduced before, satisfies this condition. We define a new unknown function 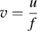 in
in 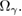 Then dividing equation (6) by
Then dividing equation (6) by 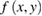 and taking into account relations (3)–(4), we obtain
and taking into account relations (3)–(4), we obtain
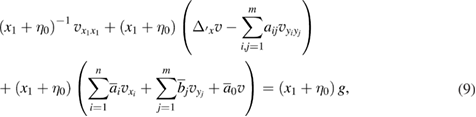


where 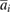 ,
, 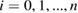 ,
,  ,
, 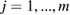 depend on the coefficients of equation (1), the function
depend on the coefficients of equation (1), the function 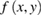 and its derivatives.
and its derivatives.
Differentiating equation (9) with respect to y m, setting 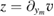 and using (11), we obtain the integro-differential equation
and using (11), we obtain the integro-differential equation
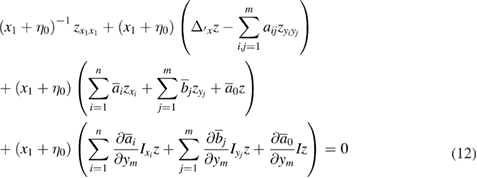
with the Cauchy data

where
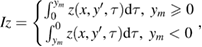
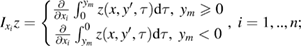
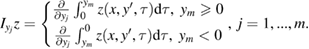
We now prove that, if  satisfies (12) and (13), then
satisfies (12) and (13), then  in
in  .
.
From (12), we obtain
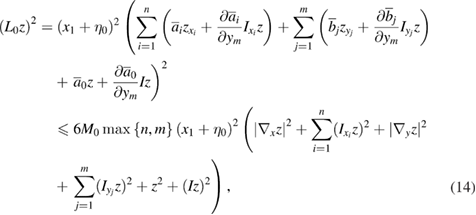
where the constant M0 > 0 depends on the coefficients aij, ak, bj ,  and
and 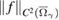 . Here we shall use the following lemma, whose proof is given in appendix.
. Here we shall use the following lemma, whose proof is given in appendix.
Lemma 1. The following relations hold:
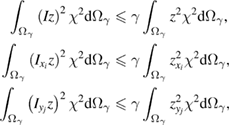
where 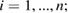
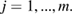
By lemma 1, we can write inequality (14) in the following form
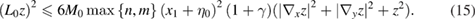
On the other hand, by proposition 1, we can write
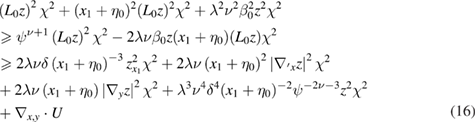
for 
 . From (15) and (16), it follows that
. From (15) and (16), it follows that
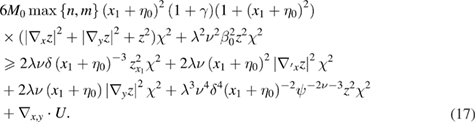
Integrating inequality (17) in  and using the fact that
and using the fact that  , we have
, we have
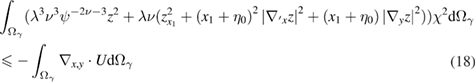
for 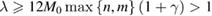 and
and  . Taking into account condition (3) and inequality (8), from (18) we can write
. Taking into account condition (3) and inequality (8), from (18) we can write
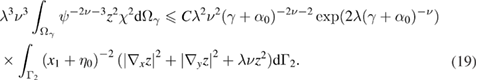
Now let us choose small  Then we see that
Then we see that 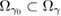 and
and
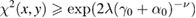
in  Hence inequality (19) implies that
Hence inequality (19) implies that
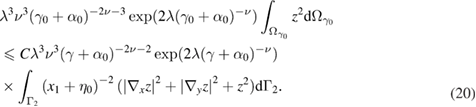
Since  and
and
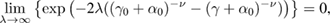
passing to the limit as  in (20), we conclude that
in (20), we conclude that
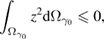
which means that 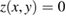 in
in 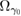 . Since
. Since 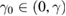 is an arbitrary number,
is an arbitrary number, 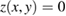 in
in 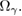
Varying the point 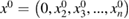 of the plane x1 = 0, we establish that z = 0 on
of the plane x1 = 0, we establish that z = 0 on 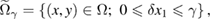 that is,
that is, 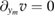 on
on  . Then from equation (9) and by condition (11) we conclude that
. Then from equation (9) and by condition (11) we conclude that  on
on 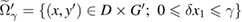 , where
, where 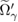 is the projection of
is the projection of 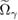 in
in 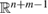 . Repeating the same argument leads to z = 0 in
. Repeating the same argument leads to z = 0 in  and
and 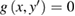 on
on 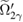 . Thus, continuing in this way we complete the proof.
. Thus, continuing in this way we complete the proof.
4. The proof of proposition 1
In the proof of proposition 1, we shall use two lemmata. The first of them is lemma 2, the proof of which is technical and lengthy, and we postpone it to appendix.
Lemma 2. Under the hypothesis of proposition 1, there exists a constant 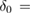
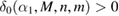 such that for any
such that for any 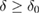 and
and 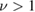 , there exists a positive number
, there exists a positive number  such that for all
such that for all  and for all
and for all  the following inequality is valid
the following inequality is valid

where 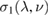 is a polynomial in
is a polynomial in  and
and  of degree 6, and the term
of degree 6, and the term 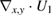 is given in the proof explicitly.
is given in the proof explicitly.
In (21), the sign of the term 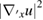 is minus and therefore we need to perform another estimation:
is minus and therefore we need to perform another estimation:
Lemma 3. The following equality holds for any function 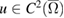 :
:
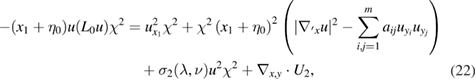
where  is a polynomial in
is a polynomial in 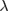 and
and  of degree 4. Moreover, the term
of degree 4. Moreover, the term 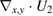 is given as follows
is given as follows
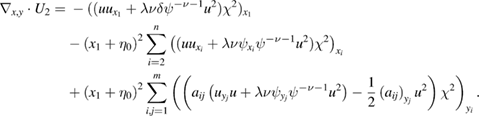
Lemma 3 can be proved easily by direct calculations and we omit the proof here.
We now proceed to the completion of the proof of proposition 1. We multiply equality (22) by  and add to inequality (21) to have
and add to inequality (21) to have

for 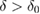 ,
,  where
where 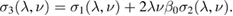
Setting 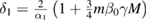 and using the inequality
and using the inequality
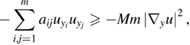
we can estimate the coefficient of  in (23):
in (23):
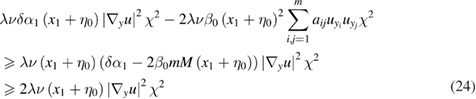
for 
As for the coefficient of u2, we can write 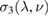 in the form
in the form

where the expressions 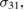
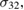
 depend on
depend on 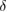 ,
, 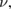 aij and
aij and  . Since the functions aij and
. Since the functions aij and 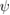 are bounded in the space
are bounded in the space 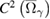 , it can be seen that the function
, it can be seen that the function 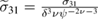 is bounded uniformly with respect to
is bounded uniformly with respect to  and
and 
 , M1 > 0. Then, noting that
, M1 > 0. Then, noting that  we have
we have
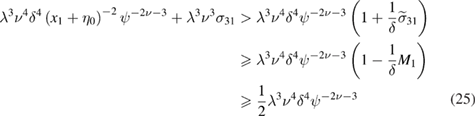
for  . It is obvious that the functions
. It is obvious that the functions 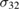 and
and  are also bounded on
are also bounded on 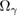 for fixed
for fixed  ,
,  , that is, there exist constants M2, M3 > 0 such that
, that is, there exist constants M2, M3 > 0 such that 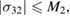
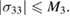 Hence, by using (25), we obtain
Hence, by using (25), we obtain
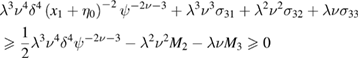
for  which yields
which yields
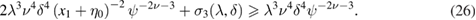
Consequently, inequalities (23), (24) and (26) imply that

for 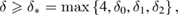
 and
and 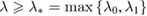 , where
, where 
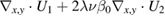 . It is easy to see that the vector function U satisfies relation (8). Thus the proof of proposition 1 is completed.
. It is easy to see that the vector function U satisfies relation (8). Thus the proof of proposition 1 is completed.
Acknowledgments
The second author was supported by Grant-in-Aid for Scientific Research (S) 15H05740 of Japan Society for the Promotion of Science and by The National Natural Science Foundation of China (no. 11771270, 91730303). This work was prepared with the support of the "RUDN University Program 5-100".
Appendix A.: Proof of lemma 1
Let K be the part of the domain  produced by the plane y m = 0 and let
produced by the plane y m = 0 and let  be the distance between the boundary point
be the distance between the boundary point 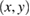 of
of  and the point
and the point 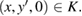
Since  is monotone with respect to y m, we have
is monotone with respect to y m, we have
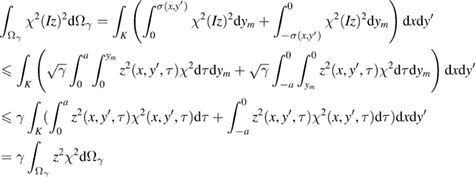
for  . Here we used the relations
. Here we used the relations
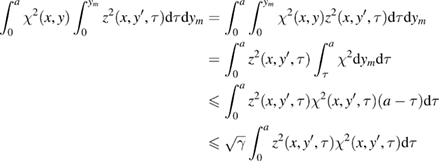
and
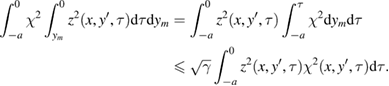
Similarly we can prove the rest part.
Appendix B.: Proof of lemma 2
The proof is based on the method described in section 4 of chapter IV in [16].
We introduce a new function
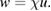
Then using the relations
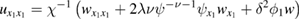
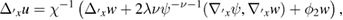
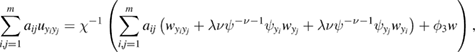
we obtain
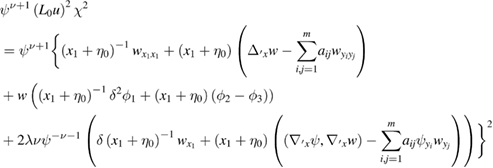
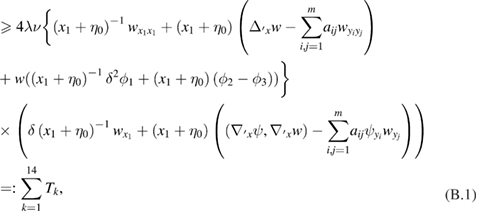
where we set
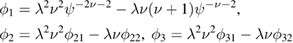
and

Here we set 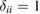 and
and  if
if 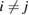 . Noting that
. Noting that 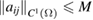 ,
,  ,
, 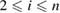 and
and  ,
, 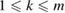 in
in 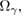 we estimate the terms Ti,
we estimate the terms Ti,  as follows:
as follows:
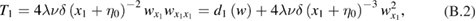
where 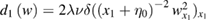 ;
;
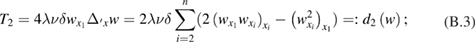
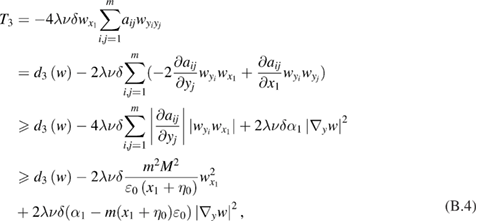
where 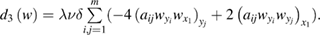
Next
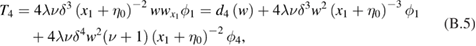
where  and we set
and we set
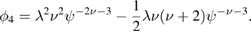
We have
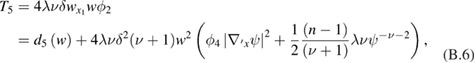
where 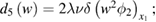

where 
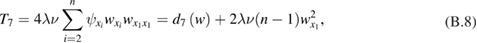
where 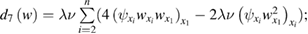
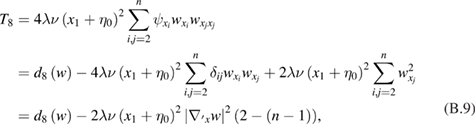
where 
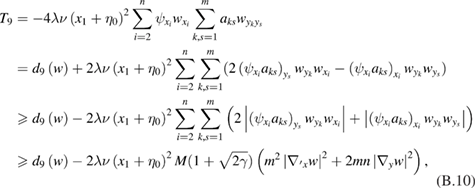
where 
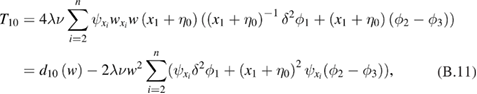
where 
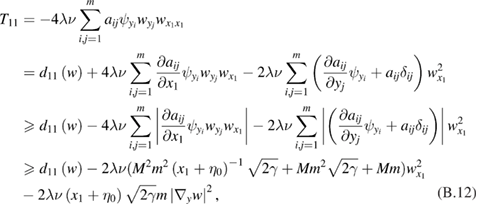
where 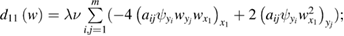
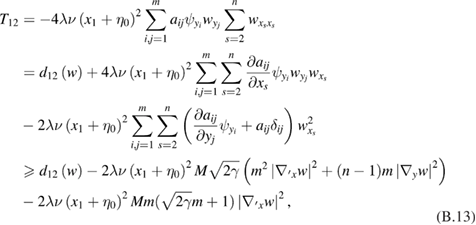
where 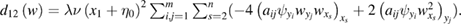
Similarly to (B.10) we obtain
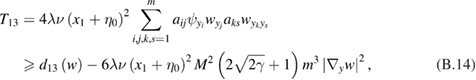
where 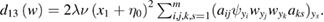
Finally we obtain
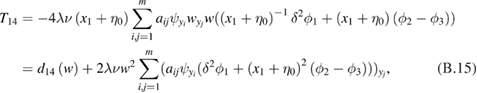
where 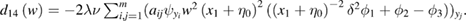
Then by relations (B.2)–(B.15), we can write
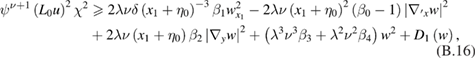
where
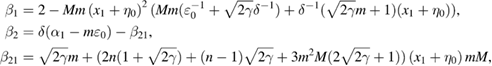
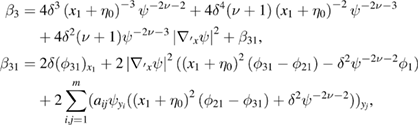
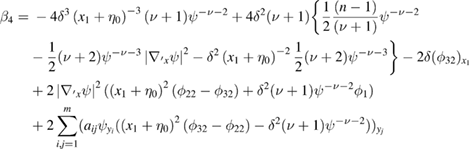
and 
Now we shall evaluate the expressions 
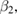

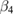 in (B.16), respectively.
in (B.16), respectively.
Since 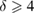 ,
, 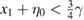 and
and 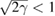 by (7), we obtain
by (7), we obtain
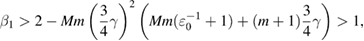
which implies
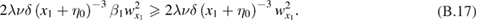
Next, by choosing  and setting
and setting 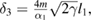 we see that
we see that

for 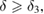 where
where 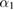 is the constant in (5) and
is the constant in (5) and
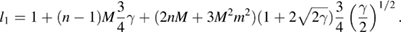
It is clear that
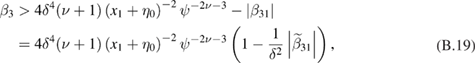
where  Since the functions aij and
Since the functions aij and 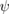 are bounded in the space
are bounded in the space 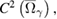 the function
the function 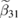 is bounded uniformly with respect to
is bounded uniformly with respect to  and
and 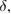 that is there exists a number M4 > 0 such that
that is there exists a number M4 > 0 such that 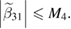 Then
Then

for 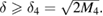 On the other hand,
On the other hand, 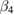 is also bounded on
is also bounded on 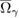 for fixed
for fixed 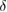 and
and  , say,
, say,  , M5 > 0, then by (B.20) we obtain
, M5 > 0, then by (B.20) we obtain
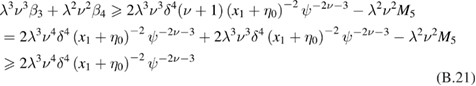
for 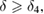
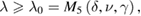

Thus, inequalities (B.17)–(B.21) lead to
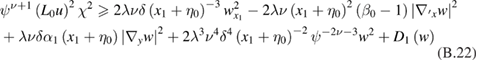
for 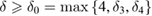 ,
,  ,
, 
Finally, returning to the original function 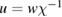 in (B.22), we obtain (21), where
in (B.22), we obtain (21), where
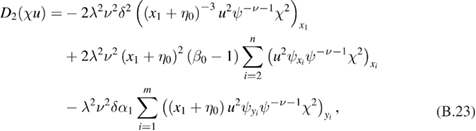
and  Thus the proof of lemma 2 is completed.
Thus the proof of lemma 2 is completed.