Abstract
This paper studies the oscillation properties of a simple pendulum immersed in a viscous liquid. A nonlinear equation of motion for the pendulum is first established by considering both viscous damping and quadratic damping, and solved by using the improved averaging method. Then a relationship between the oscillation period and the viscosity of liquid is derived. The experimental data obtained are consistent with the theoretical prediction, which also suggests a new approach to measure the liquid viscosity. We believe that this paper may be helpful for broadening teaching content for the simple pendulum and for better understanding the vibration of an object in liquids in some applications.
Export citation and abstract BibTeX RIS
1. Introduction
What happens to the oscillation period when a simple pendulum is immersed in a liquid? No answer is given in traditional textbooks, which only describe the pendulum in the air. However, this issue is related to the breadth of teaching content in classical mechanics. Furthermore, taking the pendulum as a model of an object may be helpful to understand the oscillations of the object in viscous liquids. In fact, these oscillations concern various applications in industry and the military, such as the ultrasonic cavitation in chemical engineering and medical diagnostic [1], the vibration characteristics of ship hull in shipbuilding projects [2, 3], the damper in shock mitigation systems [4, 5], the noise reduction for submarine shells [6, 7], etc. Thus, an exhaustive study of oscillation properties of a simple pendulum in liquids, in view of both teaching and applications, is essential.
The oscillation of an object in liquid is mainly affected by both the viscous damping and the quadratic damping. The former is directly proportional to the relative velocity of the object to the liquid, whereas the latter is proportional to the square of relative velocity [3, 8, 9]. The mainstream research on the oscillation phenomenon falls into two categories. For category one, the object moves slowly or the liquid viscosity is dominant, and thus, only viscous damping is considered [3]. For category two, the object moves fast or the viscosity is too small to be considered, and thus, only the quadratic damping is considered in this case, such as the vibration of a pendulum in water [11]. In general, however, viscous damping and quadratic damping exist simultaneously. But few reports are published on the oscillation affected by both dampings, perhaps due to the complexity of damping and nonlinearity of the equation of motion.
In this paper, we investigate the oscillation property of a pendulum immersed in viscous liquids. We first solve the nonlinear equation of motion by considering the two kinds of damping. Afterwards, we deduce the relation of the oscillation period to the liquid viscosity from the solution of the equation. Finally, we perform a series of experiments to compare and contrast our theoretical prediction.
2. Theoretical analysis
When an object moves slowly in a liquid, it is subjected to a viscous resistance. The viscous resistance is directly proportional to the velocity of the object, i.e. , or
, or , where
, where  is the viscous resistance coefficient. For example, the viscous resistance of a spherical object which moves slowly in a liquid is governed by the following Stokes' formula [8, 9]
is the viscous resistance coefficient. For example, the viscous resistance of a spherical object which moves slowly in a liquid is governed by the following Stokes' formula [8, 9]

where 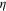 is the liquid viscosity,
is the liquid viscosity,  is the velocity of the object, and
is the velocity of the object, and  is the radius of the sphere. When an object moves fast, vortex is generated behind the object. Therefore, the pressure of the liquid in front of the object will be greater than that behind the object, which leads to quadratic resistance to the object. In this case, the resistance does not satisfy the linear Stokes' equation any more, rather it displays nonlinearity.
is the radius of the sphere. When an object moves fast, vortex is generated behind the object. Therefore, the pressure of the liquid in front of the object will be greater than that behind the object, which leads to quadratic resistance to the object. In this case, the resistance does not satisfy the linear Stokes' equation any more, rather it displays nonlinearity.
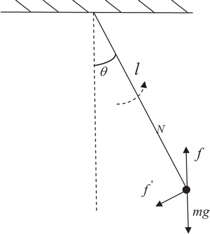
Now, let us establish the dynamic equation of a pendulum ball oscillating in a liquid. As shown in figure 1, 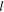 is the length of string,
is the length of string, 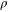 is the density of the ball,
is the density of the ball, 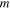 is the mass of the ball,
is the mass of the ball,  is the radius of the ball, and
is the radius of the ball, and  is the liquid density. The ball is entirely immersed in a liquid at all times. The forces acting upon the ball are the tension
is the liquid density. The ball is entirely immersed in a liquid at all times. The forces acting upon the ball are the tension  of string, the gravitational force
of string, the gravitational force 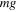 , the floating force
, the floating force 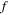 , and the liquid resistance
, and the liquid resistance 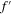 , respectively.
, respectively.
Figure 1. Forces acting upon a pendulum ball immersed in a liquid.
Download figure:
Standard image High-resolution imageAccording to the law of rotation [8], the equation of motion is the following

where  is the oscillating angle between the string and the vertical line. When
is the oscillating angle between the string and the vertical line. When 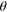 is small,
is small,  , and the equation (2) simplifies to
, and the equation (2) simplifies to

where 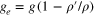 represents the equivalent acceleration of gravity. In addition, the liquid resistance
represents the equivalent acceleration of gravity. In addition, the liquid resistance 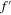 can be reasonably supposed to be
can be reasonably supposed to be

where  and
and 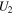 respectively stand for the viscous resistance coefficient and the quadratic resistance coefficient. Substituting (4) into (3), we get
respectively stand for the viscous resistance coefficient and the quadratic resistance coefficient. Substituting (4) into (3), we get
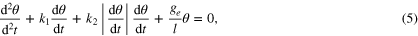
where


in which, the parameters 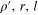 can be experimentally measured.
can be experimentally measured.
In order to investigate the variation pattern of 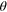 with respect to
with respect to 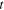 , we use MAPLE to solve equation (5) for different values of
, we use MAPLE to solve equation (5) for different values of  and
and 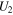 . Figure 2 shows the numerical graphic output with the parameters:
. Figure 2 shows the numerical graphic output with the parameters: 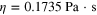 ,
,  ,
, 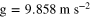 ,
, 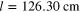 ,
,  ,
, 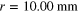 , and
, and 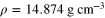 .
.
Figure 2. Relationship of oscillating angle  to time
to time  .
.
Download figure:
Standard image High-resolution imageIn figure 2, the motion of the pendulum ball in liquids is a quasi-periodic oscillation of non-exponential attenuation, and the period has a small fluctuation. The attenuation of oscillating amplitude gets faster as  or
or  becomes larger. Moreover, when the damping coefficient is over a threshold, we would observe over-damped phenomenon.
becomes larger. Moreover, when the damping coefficient is over a threshold, we would observe over-damped phenomenon.
To further obtain an analytic expression, we apply the improved averaging method [10, 11] to analytically solve the nonlinear differential equation (5). Rewriting it as

where  is the inherent frequency and
is the inherent frequency and

Let us assume the solution of equation (8) is in the form

and denote 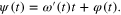 Then
Then
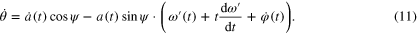
Under the assumption
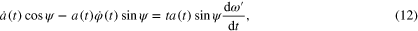
the derivative of 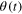 simplifies to
simplifies to

Note that the inherent frequency  is changed to
is changed to 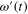 as a result of the damping here. For convenience in the following expressions, we introduce a symbol as follows:
as a result of the damping here. For convenience in the following expressions, we introduce a symbol as follows:

In this way, 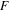 should depend on the damping coefficients
should depend on the damping coefficients 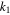 and
and 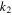 . For simplicity, we take
. For simplicity, we take  as a linear combination of
as a linear combination of 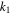 and
and  . According to the equations (6) and (7),
. According to the equations (6) and (7), 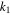 is proportional to the viscosity
is proportional to the viscosity 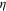 , and
, and 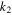 is proportional to the liquid density
is proportional to the liquid density  , therefore we may take
, therefore we may take 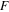 as a linear function as follows:
as a linear function as follows:

Assuming the derivative of  is small, we approximately have
is small, we approximately have

Considering equations (10) and (13), and substituting the derivative of equation (13) into equation (8), we have
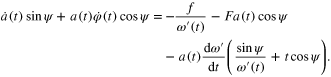
The derivative of 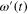 is small, therefore we have
is small, therefore we have
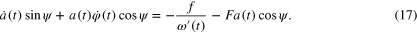
Solving the system composed of equations (16) and (17) for  and
and  , we get
, we get
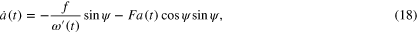
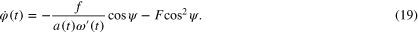
Approximating the right-hand sides of equations (18) and (19) respectively by the average values over one period of the variable 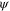 , we achieve the following equations:
, we achieve the following equations:

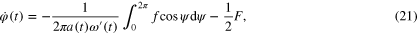
where 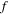 is updated as
is updated as
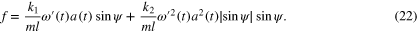
If the difference between  and
and 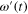 is small, then we have the following approximation
is small, then we have the following approximation

Substituting equation (22) into (20) and (21), we obtain


Integrating (25), we get 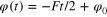 , where
, where  is a constant. Substituting
is a constant. Substituting 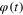 and equation (23) into (10), we have
and equation (23) into (10), we have
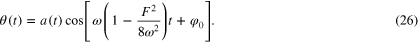
It is evident that the oscillation angle 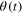 assumes a period
assumes a period

Substituting equation (15) into (27), the relationship between the period of oscillation and the viscosity is finally found to be the following:

of which the coefficients 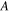 and
and 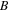 can be determined by fitting experimental data.
can be determined by fitting experimental data.
Now let us discuss the situation of  . In this case, only viscous damping exists and equation (5) simplifies to
. In this case, only viscous damping exists and equation (5) simplifies to

where  . A well-known analytical solution of equation (29) is
. A well-known analytical solution of equation (29) is
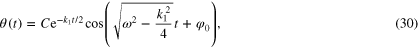
where 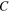 is a constant, and the oscillation angle
is a constant, and the oscillation angle  assumes a period
assumes a period

If we perform Taylor expansion for the denominator of equation (31) to the first order:

then
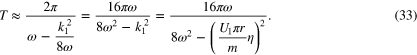
It is just in the same form as formula (28) derived by the improved averaging method.
3. Experiment and result
To validate the analytical formula (28), a series of experiments are performed to measure the oscillation period of a simple pendulum in viscous liquids. The local gravitational acceleration is first determined as  . The length of the string l = 126.30 cm, the mass of ball m = 62.305 g, the radius of ball r = 10.00 mm, the density of ball ρ = 14.874 g cm−3, and the liquid density ρ' = 1.282 ± 0.014 g cm−3. In the experiments, the mixed solution of glycerin and water is made as a liquid medium, and its viscosity
. The length of the string l = 126.30 cm, the mass of ball m = 62.305 g, the radius of ball r = 10.00 mm, the density of ball ρ = 14.874 g cm−3, and the liquid density ρ' = 1.282 ± 0.014 g cm−3. In the experiments, the mixed solution of glycerin and water is made as a liquid medium, and its viscosity 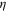 is measured by a viscometer. A heavy ball made of tungsten steel is used as a simple pendulum totally immersed in the liquid. The laboratory temperature is kept as a constant to make sure that the liquid's viscosity is not affected by the temperature. The ball periodically oscillates with a small angle and without spin. The oscillation period is measured by a photoelectric gate. To reduce the error, the experiment in one kind of liquid is repeated 14 times and then the average value of these measurements is taken as an oscillation period
is measured by a viscometer. A heavy ball made of tungsten steel is used as a simple pendulum totally immersed in the liquid. The laboratory temperature is kept as a constant to make sure that the liquid's viscosity is not affected by the temperature. The ball periodically oscillates with a small angle and without spin. The oscillation period is measured by a photoelectric gate. To reduce the error, the experiment in one kind of liquid is repeated 14 times and then the average value of these measurements is taken as an oscillation period  .
.
Note that the speed of the pendulum ball is small if the viscosity is greater than 0.3 Pa · s so that the error of the photoelectric gate becomes large; on the other hand, the quadratic resistance is much larger than the viscous resistance when the viscosity is less than 0.05 Pa · s, which makes equation of motion (5) invalid. Therefore, we choose a range of viscosity from 0.05 Pa · s to 0.3 Pa · s in ten kinds of liquids. Accordingly, the liquid density 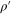 ranges from 1.268 g cm−3 to 1.295 g cm−3. The effect of change of liquid density on the oscillation period is experimentally investigated. Two groups of salt–sugar–water solutions are used as the liquids which have both the small viscosity and almost the same densities of glycerin–water solution. It is found that the effect is very small (about 1.8%), i.e.,
ranges from 1.268 g cm−3 to 1.295 g cm−3. The effect of change of liquid density on the oscillation period is experimentally investigated. Two groups of salt–sugar–water solutions are used as the liquids which have both the small viscosity and almost the same densities of glycerin–water solution. It is found that the effect is very small (about 1.8%), i.e., 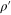 can be reasonably considered as a constant.
can be reasonably considered as a constant.
Experimental data are shown in figure 3. Fitting the data for model (28), we get the relationship between the period 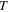 and the viscosity
and the viscosity 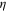 as follows:
as follows:

Figure 3. Relationship between period of pendulum and viscosity of glycerin solution.
Download figure:
Standard image High-resolution imageEvidently, the theoretical prediction is in agreement with the experimental result. Not only that, equation (34) still suggests a method to evaluate the liquid's viscosity by measuring the oscillation period of a simple pendulum when viscometers are not available or applicable.
4. Conclusion
The kinetic characteristics of a pendulum ball in a liquid with both viscous damping and quadratic damping is systematically investigated. The relation between the oscillation period and the viscosity of liquid is verified by the experiment. Our result and the underlying method may be helpful not only to enrich the content of mechanics courses but also to study the oscillation of an object in the viscous liquid in a more general setup.
Acknowledgments
This work is supported by National Innovative Experimental Projects for University Students, and the National Natural Science Foundation of China under grant No. 10975014.