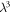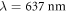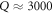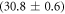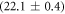Nanodiamonds in Fabry-Perot cavities: a route to scalable quantum computing

To first order, crystals like diamond should be clear because their electrons are all bound — either in small orbits around the nucleus, or in strong inter-atomic bonds. However, defects in the lattice perforce give rise to extra degrees of freedom for electrons, which in turn leads to a rich array of absorption and luminescence properties. Such defects are called colour centres, and they give us the beauty of coloured diamonds.

Amongst the over 500 identified colour centres in diamond [1], the negatively-charged nitrogen-vacancy (NV) colour centre in diamond is unprecedented for its quantum mechanical properties. It has an electronic spin triplet ground state, with zero-field splitting of 2.88 GHz between the spin 0 and spin ±1 states. Because of the relatively low concentration of nuclear spins at natural carbon abundance and the large phonon frequency relative to this splitting, these states are relatively long-lived even at room temperature. Isotopic enrichment of the carbon extends the coherence times to the millisecond regime [2]. Perhaps more remarkable than the quantum coherence time is the fact that the spin states can be optically read out and initialised [3]. The optical readout channel on isolated centres led to a raft of beautiful demonstrations, including the creation of small quantum registers [4], and nanoscale sensing modalities: magnetometry [5–7], electrometry [8], thermometry [9–11] and quantum decoherence microscopy [12, 13]. Because the optical lifetime is short with high quantum efficiency, single photon emission for quantum cryptography has also been demonstrated [14].

A solid-state, room temperature, bio-compatible qubit with single-photon emission properties is such a heady prospect that NV centres have been the target of more than their fair share of proposals. So it is necessary to address what is wrong with NV diamond for quantum computation to appreciate the beautiful work reported by Johnson et al [15].

The optical channel was quickly identified as being the most promising way to scalably entangle multiple NV centres. Lukin and Hemmer [16] proposed a system with multiple NV centres in the same cavity, which was later developed in [17]. Such proposals incorporate cavity quantum electro-dynamics as a way of inducing optically mediated NV-NV, and hence qubit-qubit, interactions, and so the cavity acts as a form of long-range bus for quantum interactions. More recent proposals have shown that cavity QED can be used in the formation of topological quantum computers through 'modules' [18–20] or other topologies [26] with individual NV-cavity systems connected by optical waveguides.

The problem with combining diamond with cavities is that it is difficult. In terms of optical properties, at first glance the NV centre seems very attractive for quantum optics. The spontaneous emission rate for NV is 12 ns (longer for nano diamonds) with main emission at 637 nm. However the fluorescence from NV has a serious problem. The 637 nm emission is the zero phonon line emission, i.e. direct radiative relaxation from the excited ³E state to the ground ³A state. At room temperature this emission corresponds to only 4% of the total emission, with the rest going into broad phononic sidebands that stretch for around 100 nm — much larger than both the homogeneous and inhomogenous broadening of the transition. Operating at cryogenic temperatures helps somewhat by sharpening the phononic structure and increasing the probability for emission into the zero phonon line. One of the key approaches to mitigating the phononic structure is to enhance the zero phonon line with an optical cavity [21].

When looking at combining cavities with diamond, two broad approaches have been used. The first is to use solid-state structures like photonic crystal or whispering gallery mode resonators. Both monolithic diamond and hybrid (diamond with a more mature photonic material) approaches have been used, see [22] for a recent review. Such structures promise intimate coupling between the emitters and the field mode, and the potential for the smallest achievable mode volumes. The problem is that the properties are set by fabrication and tuning is difficult, although not impossible. The alternative pursued here, is external Fabry-Perot cavities, which have the advantage of great flexibility in terms of alignment and spectral tuning at the potential cost of larger mode volume.

Conceptually, the Fabry-Perot cavities shown by Johnson et al [15] are no different from the cavities used in lasers and undergraduate laboratories. They comprise two mirrors, one flat and the other curved, with the emitter positioned on the flat mirror with the curved mirror positioned in three dimensions to maximise the spatial and spectral collection of emission into the mode. The complexity arises because the two mirrors need to be as close as possible; here they were separated by 1.1 μm, with radius of curvature of the curved mirror being 7.6 μm. Nevertheless, such technical difficulties have been surmounted, and some rather beautiful results have been obtained recently [23–25].

What the Oxford and Grenonble-Alpes team has demonstrated is quite remarkable. Their Fabry-Perot cavity has a mode volume of 4.7 ${\lambda }^{3}$, where $\lambda =637\;{\rm{nm}}$ is the wavelength of the zero-phonon line emission from the NV centre, and a cavity $Q\approx 3000$. This combination is enough to enhance the spontaneous emission rate (decrease the lifetime) of an identified NV centre from $(30.8\pm 0.6)$ ns to $(22.1\pm 0.4)$ ns (the lifetime of NV centres in nanodiamonds is typically longer than for centres in bulk single-crystal diamond). Diamond-integrated fibre cavities are progressing rapidly and also have the potential for far higher cavity Q than demonstrated here [23–25].

All of the the steps in this work appear to be scalable and tuneable. So that this demonstration of a single NV nanodiamond coupled to a cavity should be able to be repeated to make multiple coupled NV-cavity systems to realise the architectures mentioned above. The relatively large cavity design also should enable integration of the cavity structure with microwave lines for control of the NV ground state, which the authors have not yet demonstrated.

Temperature has always been an important factor for diamond quantum computation. Despite the demonstration of room-temperature quantum coherence, optical approaches to scalability have all required cryogenic operation below 10 K [27]¹ . Remarkably, the work by Johnson et al is at 77 K [15]. This greatly reduces the cost and improves the accessible cooling power by enabling liquid nitrogen operation of any future quantum scheme if indistinguishable photons can be realised in such a setup.

The question of whether the right quantum material is nanodiamond or diamond membranes still needs to be addressed. Nanodiamonds are cheap and relatively easy to integrate into the cavity, as is shown here. However both emission and ground state lifetime of NV in nanodiamonds appear to be significantly worse than in large single-crystal diamonds. Additionally, alignment of the nanodiamond, and hence the orientation of the NV with respect to the cavity mode is a random process. Diamond membranes remove some of the random alignment problems and are rapidly increasing in quality. Demonstration of coupling between NV in a 10 μm diamond membrane and a fibre microcavity was shown in [25], and single crystal diamond membranes can be made with thicknesses down to 200 nm [29].

Diamond containing the nitrogen-vacancy colour centre is proving to be one of the most versatile platforms for quantum information processing. This latest movement towards open cavities seems to show the flexibility and tunability that is required for a scalable diamond quantum computer, and one cannot help but feel optimistic about the prospects for quantum diamond.

Acknowledgments