Abstract
The length of single-walled carbon nanotubes (SWCNTs) is an important metric for the integration of SWCNTs into devices and for the performance of SWCNT-based electronic or optoelectronic applications. In this work we propose a rather simple method based on electric-field induced differential absorption spectroscopy to measure the chiral-index-resolved average length of SWCNTs in dispersions. The method takes advantage of the electric-field induced length-dependent dipole moment of nanotubes and has been verified and calibrated by atomic force microscopy. This method not only provides a low cost, in situ approach for length measurements of SWCNTs in dispersion, but due to the sensitivity of the method to the SWCNT chiral index, the chiral index dependent average length of fractions obtained by chromatographic sorting can also be derived. Also, the determination of the chiral-index resolved length distribution seems to be possible using this method.
Export citation and abstract BibTeX RIS
Single-walled carbon nanotubes (SWCNTs) have a unique structure-property correlation which allows the nanotube's chiral index and hence its diameter to be determined by spectroscopic methods such as absorption, fluorescence or Raman spectroscopy [1]. In contrast, the length of a carbon nanotube has little influence on its optical properties and therefore a direct spectroscopic access to the SWCNT length beyond 100 nm is not available [2, 3], Consequently, methods that were proven to be very accurate in gauging nano-scale and micro-scale dimensions such as transmission electron microscopy, scanning electron microscopy and atomic force microscopy (AFM) have been applied to measure the length of a nanotube or the length distribution of a nanotube ensemble. In particular the AFM method has evolved as a kind of standard approach to measure nanotube length distributions [4, 5], even though the characterization method requires specific substrates, and additional preparation steps and is associated with uncertainties due to nanotube bundling on surfaces or selective adsorption. Therefore, the search for new SWCNT length measurement methods is ongoing, and especially the field of SWCNT liquid phase sorting would benefit from a fast and practical in situ length characterization method. In recent years, a range of in situ methods have been explored such as dynamic light scattering [6–9], electrospray differential mobility analysis [10], diffusional trajectory [11], shear-aligned photoluminescence anisotropy [12] and analytical ultracentrifugation [13]. The most advanced in situ measurement of the length distribution of dispersed SWNCTs has been reported by the Weisman group who introduced the length analysis by nanotube diffusion method (LAND) [11]. The LAND method yields length distributions very similar to the comparative ex situ AFM method, but unfortunately it requires a dedicated fluorescence microscopy setup that is not available to all researchers in the field. Hence we wanted to develop an in situ method that is based on standard equipment and easy to implement. Moreover we decided to refrain from measuring the length distribution, but rather aimed at developing a method for measuring the average length, which is sufficient for many practical purposes. This led us to a rather simple method based on electric-field induced differential absorption spectroscopy (EFIDAS), which allows determining directly the average length of SWCNTs in dispersions and provides chiral-index-resolution as an added value. The method takes advantage of the electric-field induced length-dependent dipole moment of nanotubes. In fact, electric-field induced alignment of SWCNTs in dispersion is not new and has been reported before [14–16]. However previous works in aqueous media could only resolve alignment of bundles of SWCNTs and not of well dispersed individual SWCNTs. Using a low-k solvent instead of water, it is in fact possible to resolve the field alignment of individually dispersed SWCNTs as we will show in this work. Moreover, since we have used dispersions with only a small number of different SWCNT chiral indices, it was possible to resolve the chiral index dependent average length. Besides opening a new approach for fast direct length mapping of dispersed SWCNTs, our method also gives novel insights into the concept of surfactant-induced nanotube conductance which was introduced some time ago to explain dielectrophoresis of semiconducting SWCNTs [17]. Furthermore simulations will show that the determination of the chiral-index resolved length distribution seems to be possible.
Results and discussion
First we introduce the concept of differential absorption and its dependence on the electric field strength, on the SWCNT diameter and on the SWCNT length, before going on to discuss the experimental results. We start with the derivation of the electric-field induced differential absorption by considering the optical absorption of carbon nanotubes in between two parallel linear polarizers 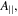 modeled as [18, 19]
modeled as [18, 19]
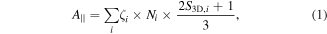
where  denotes the different (n, m) SWCNT species in solution with absorption coefficient
denotes the different (n, m) SWCNT species in solution with absorption coefficient  and counting number
and counting number  stands for the three-dimensional nematic order parameter [19], which has a lower-limit of
stands for the three-dimensional nematic order parameter [19], which has a lower-limit of  for a completely disordered phase,
for a completely disordered phase,  for a weakly ordered phase and an upper-limit of
for a weakly ordered phase and an upper-limit of  corresponding to a perfectly ordered phase. For SWCNTs with diameter
corresponding to a perfectly ordered phase. For SWCNTs with diameter  and length
and length  exposed to a homogeneous electric field
exposed to a homogeneous electric field 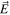 with angular frequency
with angular frequency 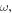 the nematic order parameter can be expressed as
the nematic order parameter can be expressed as  with the complex dielectric functions
with the complex dielectric functions  and
and 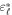 of the nanotubes and the solvent medium, respectively [20] (see also supporting information).
of the nanotubes and the solvent medium, respectively [20] (see also supporting information).
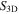 is a direct measure of the orientation of SWCNTs under an external electric field, provided that the absorption coefficient
is a direct measure of the orientation of SWCNTs under an external electric field, provided that the absorption coefficient  in equation (1) is constant under the applied electric field. For our experiment this assumption is valid when considering the electro-absorption experiments of surface-pinned SWCNTs by Izard et al [21, 22]. Izard's data shows that upon applying an electric field of 12.5 kV cm−1 the changes of
in equation (1) is constant under the applied electric field. For our experiment this assumption is valid when considering the electro-absorption experiments of surface-pinned SWCNTs by Izard et al [21, 22]. Izard's data shows that upon applying an electric field of 12.5 kV cm−1 the changes of 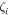 are on the order of order of 10−4, whereas in our experiments with dispersed SWCNTs we observe changes in
are on the order of order of 10−4, whereas in our experiments with dispersed SWCNTs we observe changes in 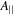 on the order of 10−1 at an electric field of 2.5 kV cm−1, as described later. Thus in our experiments
on the order of 10−1 at an electric field of 2.5 kV cm−1, as described later. Thus in our experiments  is predominantly determined by the orientation of SWCNTs as reflected by
is predominantly determined by the orientation of SWCNTs as reflected by 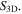 Also transverse polarized transitions can be ignored due to a large depolarization effect in SWCNTs [23]. The electric-field induced, differential absorption
Also transverse polarized transitions can be ignored due to a large depolarization effect in SWCNTs [23]. The electric-field induced, differential absorption 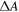 is then simply the relative difference of the optical absorption with and without an electric field. Since
is then simply the relative difference of the optical absorption with and without an electric field. Since 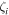 is not electric field dependent,
is not electric field dependent,  is only proportional to
is only proportional to 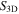
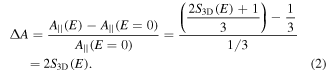
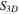 can be expressed in terms of the alignment angle
can be expressed in terms of the alignment angle  as
as
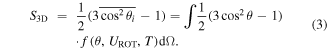
The bar indicates the mean value,  is measured between the long-axis of the nanotube and the electric field direction, which is also the polarization direction of the incident light in our experiment, and
is measured between the long-axis of the nanotube and the electric field direction, which is also the polarization direction of the incident light in our experiment, and 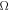 is the solid angle.
is the solid angle.  is the rotational energy of the SWCNT in the electric field and
is the rotational energy of the SWCNT in the electric field and  is the Boltzmann distribution function.
is the Boltzmann distribution function.  is calculated on the basis of the polarizability of SWCNTs in solution as described in detail by Blatt et al [24]. T is the temperature and set to 300 K. On this basis
is calculated on the basis of the polarizability of SWCNTs in solution as described in detail by Blatt et al [24]. T is the temperature and set to 300 K. On this basis  and
and 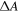 can be calculated as a function of
can be calculated as a function of 
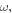
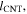
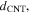

 as outlined in the supporting information.
as outlined in the supporting information.  can be written as
can be written as 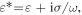 with
with 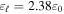 and
and 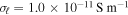 for toluene [25]. For SWCNTs,
for toluene [25]. For SWCNTs, 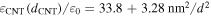 deduced from first-principles calculations of Marzari et al [26], yielding values between 35.4
deduced from first-principles calculations of Marzari et al [26], yielding values between 35.4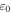 and 36.3
and 36.3 for the SWCNTs under consideration. From previous dielectrophoresis experiments
for the SWCNTs under consideration. From previous dielectrophoresis experiments  is on the order of
is on the order of 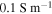 [17, 24]. It should be emphasized that the simulation contains no additional free parameter.
[17, 24]. It should be emphasized that the simulation contains no additional free parameter.
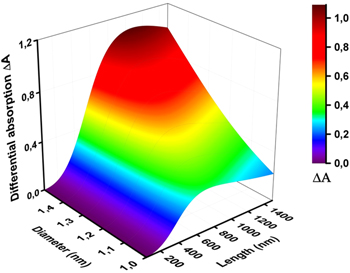
Figure 1 shows how the differential absorption of semiconducting SWCNTs in toluene depends on the nanotube length and diameter when exposed to an electric field 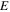 = 2.5 kV cm−1 at a frequency
= 2.5 kV cm−1 at a frequency  For SWCNTs with length up to 1.5 μm and diameter between 1–1.5 nm,
For SWCNTs with length up to 1.5 μm and diameter between 1–1.5 nm, 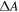 can reach values of unity and therefore is experimentally accessible. Perfect alignment would correspond to
can reach values of unity and therefore is experimentally accessible. Perfect alignment would correspond to  however this value cannot be reached due to depolarization effects, which become more pronounced for smaller diameter SWCNTs and will be discussed later. The sensitivity of
however this value cannot be reached due to depolarization effects, which become more pronounced for smaller diameter SWCNTs and will be discussed later. The sensitivity of 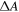 to a certain SWCNT length range can be tuned by varying
to a certain SWCNT length range can be tuned by varying 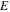 (figure S4).
(figure S4).
Figure 1. Simulation of differential absorption ΔA as a function of SWCNT diameter dCNT and length lCNT for semiconducting SWCNTs dispersed in toluene. The electric field and frequency was set to Erms = 2.5 kV cm−1 and ν = 20 kHz.
Download figure:
Standard image High-resolution imageWe will now compare the simulations with the experimental results. For measuring 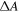 we have used the set-up shown in figure 2. The core is a cuvette with external electrodes driven by a signal generator to generate an electric field that is perpendicular to the light path and parallel to the horizontal alignment of the two linear polarizers. Light from a halogen lamp is guided through the polarizers and cuvette, and detected by a silicon CCD based spectrometer. Each measurement of
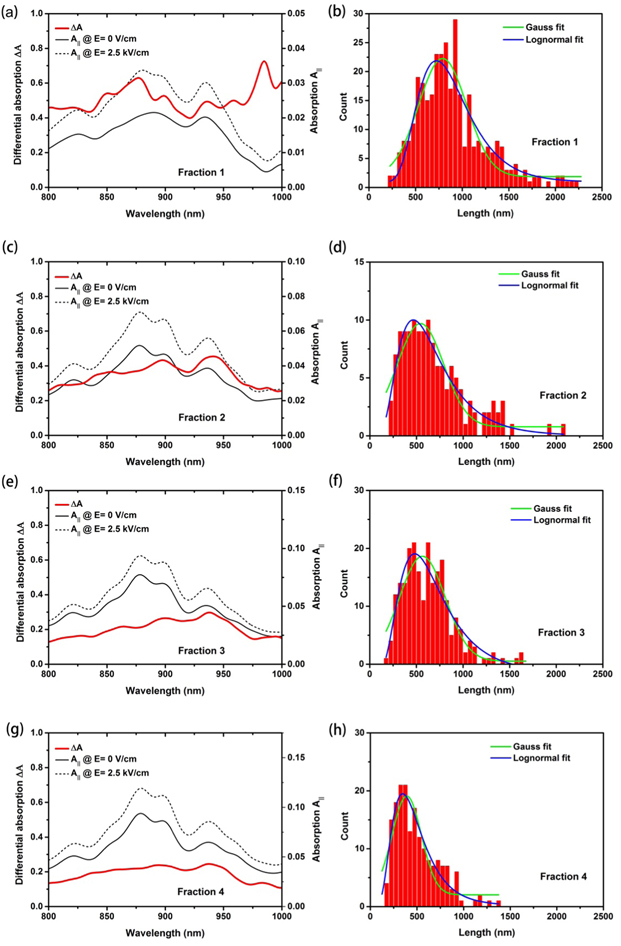
we have used the set-up shown in figure 2. The core is a cuvette with external electrodes driven by a signal generator to generate an electric field that is perpendicular to the light path and parallel to the horizontal alignment of the two linear polarizers. Light from a halogen lamp is guided through the polarizers and cuvette, and detected by a silicon CCD based spectrometer. Each measurement of  comprises a zero-field measurement and a field measurement. The signal generator supplies a 20 kHz signal with a voltage amplitude of 2380 V, resulting in an electric field strength in toluene of 2.5 kV cm−1. This field strength has been used for the simulation in figure 1. We have studied dispersions of polymer-wrapped SWCNTs in toluene containing mainly (9, 8), (10, 8), (10, 9), (11, 7), (11, 9), (11, 10) and (12, 8) SWCNTs detectable by the EFIDAS setup via their S22 transitions, as confirmed by additional absorption and photoluminescence spectroscopy (figures S2 and S3). We have measured EFIDAS with 4 fractions that were length sorted as described in [27]. For reference their length distribution was characterized by AFM. Fitting the measured length distribution to a Gaussian and lognormal distribution function, the Gaussian mean value and lognormal peak values of the fractions were determined within the given uncertainty for fraction 1 to 790 ± 18 nm and 720 ± 27 nm, for fraction 2 to 543 ± 19 nm and 465 ± 24 nm, for fraction 3 to 564 ± 19 nm and 480 ± 39 nm, and for fraction 4 to 390 ± 18 nm and 345 ± 20 nm, respectively. Hence the average length of SWCNTs decreases from fraction 1 to fraction 4, which is a consequence of the longer retention time of shorter nanotubes on the column [28]. More details about the spectroscopy and materials are contained in the methods section.
comprises a zero-field measurement and a field measurement. The signal generator supplies a 20 kHz signal with a voltage amplitude of 2380 V, resulting in an electric field strength in toluene of 2.5 kV cm−1. This field strength has been used for the simulation in figure 1. We have studied dispersions of polymer-wrapped SWCNTs in toluene containing mainly (9, 8), (10, 8), (10, 9), (11, 7), (11, 9), (11, 10) and (12, 8) SWCNTs detectable by the EFIDAS setup via their S22 transitions, as confirmed by additional absorption and photoluminescence spectroscopy (figures S2 and S3). We have measured EFIDAS with 4 fractions that were length sorted as described in [27]. For reference their length distribution was characterized by AFM. Fitting the measured length distribution to a Gaussian and lognormal distribution function, the Gaussian mean value and lognormal peak values of the fractions were determined within the given uncertainty for fraction 1 to 790 ± 18 nm and 720 ± 27 nm, for fraction 2 to 543 ± 19 nm and 465 ± 24 nm, for fraction 3 to 564 ± 19 nm and 480 ± 39 nm, and for fraction 4 to 390 ± 18 nm and 345 ± 20 nm, respectively. Hence the average length of SWCNTs decreases from fraction 1 to fraction 4, which is a consequence of the longer retention time of shorter nanotubes on the column [28]. More details about the spectroscopy and materials are contained in the methods section.
Figure 2. Schematic of the electric-field induced differential absorption spectroscopy (EFIDAS) setup. The linear polarizers are horizontally aligned (red arrows), parallel to the direction of the electric field generated across the cuvette.
Download figure:
Standard image High-resolution imageThe EFIDAS setup allows measuring  associated with the second optical transitions S22 of the dispersions. Figure 3 shows the differential absorption spectra
associated with the second optical transitions S22 of the dispersions. Figure 3 shows the differential absorption spectra  derived from the absorption spectra with and without applied electric field for the fractions 1–4 in ((a), (c), (e), (g)), next to the corresponding length distributions determined by AFM in ((b), (d), (f), (h)). First, we observe that the overall minimum and maximum of
derived from the absorption spectra with and without applied electric field for the fractions 1–4 in ((a), (c), (e), (g)), next to the corresponding length distributions determined by AFM in ((b), (d), (f), (h)). First, we observe that the overall minimum and maximum of 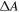 is 0.1 and 0.75, respectively, and hence within the range of the simulation. Second, we observe that
is 0.1 and 0.75, respectively, and hence within the range of the simulation. Second, we observe that  scales systematically with the SWCNTs average length, with the highest value measured for fraction 1 and the lowest for fraction 4. This observation shows that EFIDAS is sensitive to the length of SWCNTs in dispersion and shows qualitatively a dependence that is expected based on the simulation. Third, we observe that
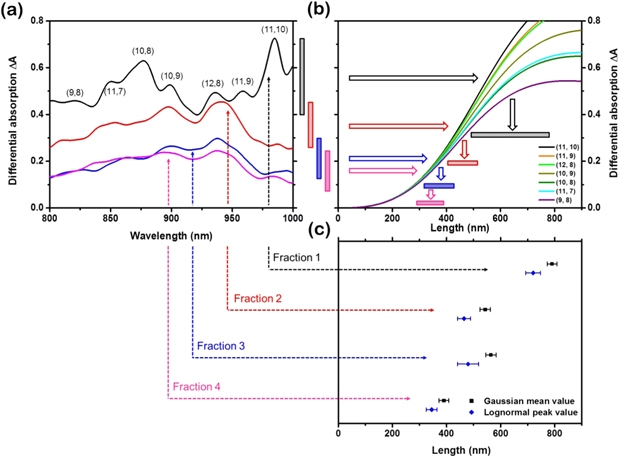
scales systematically with the SWCNTs average length, with the highest value measured for fraction 1 and the lowest for fraction 4. This observation shows that EFIDAS is sensitive to the length of SWCNTs in dispersion and shows qualitatively a dependence that is expected based on the simulation. Third, we observe that  is wavelength dependent, with maxima and minima correlating to structures in the absorption spectra. Figure 4(a) shows for fraction 1 an assignment of the peaks in
is wavelength dependent, with maxima and minima correlating to structures in the absorption spectra. Figure 4(a) shows for fraction 1 an assignment of the peaks in 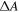 to the SWCNT chiral index based on reference absorption and photoluminescence spectra shown in the supporting information. Since concentration effects drop out in EFIDAS we can trace back the modulations in
to the SWCNT chiral index based on reference absorption and photoluminescence spectra shown in the supporting information. Since concentration effects drop out in EFIDAS we can trace back the modulations in 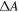 to a chiral-index dependent degree of alignment. Interestingly the modulations in
to a chiral-index dependent degree of alignment. Interestingly the modulations in 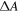 are also present in the shorter SWCNT fractions 2–4, where, according to figure 1,
are also present in the shorter SWCNT fractions 2–4, where, according to figure 1,  is no longer sensitively dependent on the SWCNT chiral index or diameter. Hence a still observable modulation of
is no longer sensitively dependent on the SWCNT chiral index or diameter. Hence a still observable modulation of 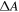 must reflect a heterogeneous length distribution across the chiral index specific SWCNT subpopulations in the different fractions.
must reflect a heterogeneous length distribution across the chiral index specific SWCNT subpopulations in the different fractions.
Figure 3. Differential absorption spectra  and absorption spectra
and absorption spectra  of semiconducting SWCNTs in toluene, measured at zero field and at Erms = 2.5 kV cm−1 and ν = 20 kHz. The data is shown for fractions 1–4 (a), (c), (e), (g) together with AFM length measurements (b), (d), (f), (h).
of semiconducting SWCNTs in toluene, measured at zero field and at Erms = 2.5 kV cm−1 and ν = 20 kHz. The data is shown for fractions 1–4 (a), (c), (e), (g) together with AFM length measurements (b), (d), (f), (h).
Download figure:
Standard image High-resolution imageFigure 4. Length determination with EFIDAS. (a) Differential absorption spectra  measured for fractions 1–5 with indicated (n, m)-specific contributions. (b) (n, m)-specific calculations of
measured for fractions 1–5 with indicated (n, m)-specific contributions. (b) (n, m)-specific calculations of 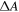 versus nanotube length lCNT. Ranges of
versus nanotube length lCNT. Ranges of 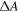 are converted into ranges of lCNT as indicated by bars and arrows. The results are compared to fitted AFM mean and peak length values for the fractions shown in (c).
are converted into ranges of lCNT as indicated by bars and arrows. The results are compared to fitted AFM mean and peak length values for the fractions shown in (c).
Download figure:
Standard image High-resolution imageWe will next extract the length of SWCNTs 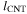 in dispersion from the differential absorption spectra
in dispersion from the differential absorption spectra 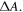 In figure 4(a) we plot
In figure 4(a) we plot 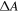 for the fractions 1–4. Ranges of
for the fractions 1–4. Ranges of  are indicated for each fraction by vertical bars. The experimental
are indicated for each fraction by vertical bars. The experimental  values can be converted into
values can be converted into  by using the simulation-derived correlation between
by using the simulation-derived correlation between 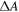 and
and 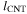 as shown in figure 4(b). The values of
as shown in figure 4(b). The values of  measured by EFIDAS are close to the AFM mean length and reproduce the trend in which higher fraction numbers have on average shorter SWCNTs (figure 4(c)). As shown, for all fractions the EFIDAS derived values fit very well to the AFM mean length.
measured by EFIDAS are close to the AFM mean length and reproduce the trend in which higher fraction numbers have on average shorter SWCNTs (figure 4(c)). As shown, for all fractions the EFIDAS derived values fit very well to the AFM mean length.
The agreement between the two methods is remarkable in particular since EFIDAS is based only on the dielectric functions of the SWCNTs and the solvent, and hence is without free parameters. Critical to the methods quantitative applicability is certainly the correlation between 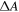 and
and  It is interesting to see that the calculated curves in figure 4(b) do not fall on top of each other. This is due to small variations in
It is interesting to see that the calculated curves in figure 4(b) do not fall on top of each other. This is due to small variations in  and
and 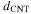 for the chiralities of interest. The influence of
for the chiralities of interest. The influence of 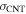 is shown in figure S5. For the simulation of figures 4(b) and 1 we have used
is shown in figure S5. For the simulation of figures 4(b) and 1 we have used  a value that gives the best fit to the AFM data and which happens to be comparable to our earlier dielectrophoresis experiments with SWCNTs in aqueous medium [24]. Previously the non-zero value of
a value that gives the best fit to the AFM data and which happens to be comparable to our earlier dielectrophoresis experiments with SWCNTs in aqueous medium [24]. Previously the non-zero value of 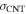 has been attributed to surface-induced conductivity mediated by ions in the aqueous double layer. For polymer-wrapped undoped SWCNTs in toluene as is the case here, we would have expected that
has been attributed to surface-induced conductivity mediated by ions in the aqueous double layer. For polymer-wrapped undoped SWCNTs in toluene as is the case here, we would have expected that  would be negligibly small. However simulations in figure S5 show that for
would be negligibly small. However simulations in figure S5 show that for 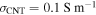 the maximum value for
the maximum value for  does not exceed 0.6, which is in contradiction with the experimental observations of (10, 8) SWCNTs, yielding a peak value of 0.63 from fraction 1. On the other hand a significantly larger value would shift the curve towards shorter
does not exceed 0.6, which is in contradiction with the experimental observations of (10, 8) SWCNTs, yielding a peak value of 0.63 from fraction 1. On the other hand a significantly larger value would shift the curve towards shorter  and lead to an underestimation of the true length when benchmarked with the AFM measurements. Since surface-induced conductivity mediated by ions can be excluded in this work,
and lead to an underestimation of the true length when benchmarked with the AFM measurements. Since surface-induced conductivity mediated by ions can be excluded in this work,  must be related to intrinsic free charge carriers present in the SWCNT. We attempt to estimate
must be related to intrinsic free charge carriers present in the SWCNT. We attempt to estimate 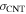 by considering 4 conduction channels that are thermally activated by
by considering 4 conduction channels that are thermally activated by 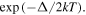 With a transport gap of Δ = 1 eV [29] and kT = 0.025 eV this would correspond to a thermally activated resistance of R ≈ 3 × 1012 Ω. Assuming
With a transport gap of Δ = 1 eV [29] and kT = 0.025 eV this would correspond to a thermally activated resistance of R ≈ 3 × 1012 Ω. Assuming 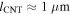 and
and  R can then formally be converted into
R can then formally be converted into 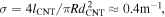 yielding the right order of magnitude. This oversimplified approach should be further developed including the influence of doping, however this would be beyond the scope of this paper.
yielding the right order of magnitude. This oversimplified approach should be further developed including the influence of doping, however this would be beyond the scope of this paper.
We continue with the length of chiral index subpopulations encoded in the EFIDAS data, and extract from figure 4(a) the differential absorption for different (n,m) species. The  values are converted into
values are converted into 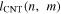 and plotted against the fraction number as shown in figure S6. The graph shows that overall the SWCNT length decreases with fraction number. Interestingly, we observe that according to EFIDAS the (n,m) length order changes from fraction 1 to fraction 2, which would mean that the elution time in GPC-based sorting not only depends on the SWCNT length but also on the chiral index. Whether the EFIDAS results are significant or whether the measurement error is underestimated remains currently unresolved. Unfortunately we cannot confirm this result by AFM measurements since AFM is not sensitive to the chiral index, but the data indicates the potential of EFIDAS for chirality-resolved length measurements in solution.
and plotted against the fraction number as shown in figure S6. The graph shows that overall the SWCNT length decreases with fraction number. Interestingly, we observe that according to EFIDAS the (n,m) length order changes from fraction 1 to fraction 2, which would mean that the elution time in GPC-based sorting not only depends on the SWCNT length but also on the chiral index. Whether the EFIDAS results are significant or whether the measurement error is underestimated remains currently unresolved. Unfortunately we cannot confirm this result by AFM measurements since AFM is not sensitive to the chiral index, but the data indicates the potential of EFIDAS for chirality-resolved length measurements in solution.
Now we discuss the dependence of EFIDAS on the electric field frequency, which is essential to understand the non-monotonic length dependence of  for small diameter nanotubes in figure 1. Effects related to the viscosity of the liquid can be ignored since we measure
for small diameter nanotubes in figure 1. Effects related to the viscosity of the liquid can be ignored since we measure  under steady-state conditions. The steady state is reached within seconds after switching on the electric field, as shown in figure S7. Also nanotube-nanotube interaction can be ignored since the nanotube concentration is ∼0.10 tube μm–3 as determined from the absorption in combination with the average nanotube length. As explained before,
under steady-state conditions. The steady state is reached within seconds after switching on the electric field, as shown in figure S7. Also nanotube-nanotube interaction can be ignored since the nanotube concentration is ∼0.10 tube μm–3 as determined from the absorption in combination with the average nanotube length. As explained before,  is calculated from the rotational energy
is calculated from the rotational energy 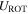 using equations (2) and (3).
using equations (2) and (3).  is defined as the integral over the time-averaged torque
is defined as the integral over the time-averaged torque  imposed on the SWCNTs in the oscillating field (equation (4)), whereas
imposed on the SWCNTs in the oscillating field (equation (4)), whereas 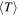 is proportional to the difference of the real parts of the longitudinal and transverse Clausius–Mossotti factors
is proportional to the difference of the real parts of the longitudinal and transverse Clausius–Mossotti factors  and
and 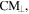 respectively (equation (5)). The Clausius–Mossotti factors contain the frequency-dependent dielectric functions of the CNT and the solvent (equation (6)):
respectively (equation (5)). The Clausius–Mossotti factors contain the frequency-dependent dielectric functions of the CNT and the solvent (equation (6)):
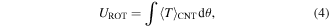
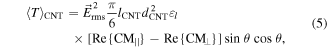

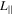 and
and 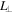 are the longitudinal and transverse depolarization factors, respectively, with
are the longitudinal and transverse depolarization factors, respectively, with 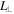 = 1/2 and
= 1/2 and ![${L}_{| | }={d}_{{\rm{C}}{\rm{N}}{\rm{T}}}^{2}/{l}_{{\rm{C}}{\rm{N}}{\rm{T}}}^{2}[\mathrm{ln}(2{l}_{{\rm{C}}{\rm{N}}{\rm{T}}}/{d}_{{\rm{C}}{\rm{N}}{\rm{T}}})-1]$](https://content.cld.iop.org/journals/0957-4484/27/37/375706/revision1/nanoaa3360ieqn112.gif) [17, 30]. The expressions can be simplified for semiconducting SWCNTs since
[17, 30]. The expressions can be simplified for semiconducting SWCNTs since  and
and 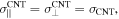 as outlined by Blatt et al [24]. Figure S8(a) shows that
as outlined by Blatt et al [24]. Figure S8(a) shows that 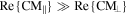 throughout our simulation space. Hence the frequency dependence of
throughout our simulation space. Hence the frequency dependence of  and thus of
and thus of 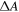 is entirely determined by
is entirely determined by  In figure 5(a) we have plotted
In figure 5(a) we have plotted 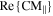 as a function of field frequency and SWCNT length for dCNT = 130 nm. The data shows that
as a function of field frequency and SWCNT length for dCNT = 130 nm. The data shows that  for ν < 105 Hz. Therefore,
for ν < 105 Hz. Therefore, 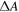 reaches sizable values only for ν < 105 Hz as shown in figure 5(b). The experimental frequency ν = 2 × 104 Hz is hence a good choice for EFIDAS based length measurements of semiconducting SWCNTs in toluene, although larger
reaches sizable values only for ν < 105 Hz as shown in figure 5(b). The experimental frequency ν = 2 × 104 Hz is hence a good choice for EFIDAS based length measurements of semiconducting SWCNTs in toluene, although larger  values are expected for lower frequencies as shown in figure 5(b). A practical measure for selecting an appropriate field frequency is the so-called Maxwell–Wagner relaxation time
values are expected for lower frequencies as shown in figure 5(b). A practical measure for selecting an appropriate field frequency is the so-called Maxwell–Wagner relaxation time  which accounts for the characteristic time scale of charge accumulation at the interface of two materials. For SWCNTs modeled as prolate ellipsoids [24, 30, 31], the relevant longitudinal Maxwell–Wagner relaxation
which accounts for the characteristic time scale of charge accumulation at the interface of two materials. For SWCNTs modeled as prolate ellipsoids [24, 30, 31], the relevant longitudinal Maxwell–Wagner relaxation  is given by
is given by 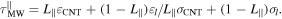 The corresponding Maxwell–Wagner relaxation frequency
The corresponding Maxwell–Wagner relaxation frequency 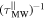 decreases with the SWCNT length and increases with the SWCNT diameter, as shown in figure S8(b), and can be traced back to the diameter and length dependence of
decreases with the SWCNT length and increases with the SWCNT diameter, as shown in figure S8(b), and can be traced back to the diameter and length dependence of 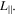 A necessary condition for effective EFIDAS measurements is fulfilled if
A necessary condition for effective EFIDAS measurements is fulfilled if  Also the non-monotonic length dependence of
Also the non-monotonic length dependence of 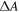 for small diameter semiconducting SWCNTs in figure 1 is due to the length and diameter dependence of
for small diameter semiconducting SWCNTs in figure 1 is due to the length and diameter dependence of  and Re{CM||}.
and Re{CM||}.
Figure 5. Simulations of (a) the real part of the longitudinal Clausius–Mossotti factor Re CM|| and (b) the differential absorption 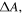 as a function of field frequency ν and nanotube length lCNT. Simulations consider semiconducting SWCNTs with diameter dCNT = 1.30 nm, dispersed in toluene and exposed to an electric field Erms = 2.5 kV cm−1. The horizontal dashed line in (a) indicates the field frequency used for the EFIDAS measurements.
as a function of field frequency ν and nanotube length lCNT. Simulations consider semiconducting SWCNTs with diameter dCNT = 1.30 nm, dispersed in toluene and exposed to an electric field Erms = 2.5 kV cm−1. The horizontal dashed line in (a) indicates the field frequency used for the EFIDAS measurements.
Download figure:
Standard image High-resolution imageA limitation of EFIDAS is that the method does not work for semiconducting SWCNTs in aqueous surfactant solution which we discuss now and show experimental results. The conductivity of water with 1 wt% SDS is 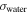 = 0.1 S m−1, and 10 orders of magnitude larger compared to toluene. This large conductivity of the solution in combination with the dielectric constant of water
= 0.1 S m−1, and 10 orders of magnitude larger compared to toluene. This large conductivity of the solution in combination with the dielectric constant of water 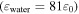 yields for the same applied voltage amplitude of Vrms = 2380 V an electric field of only Erms = 1.2 × 10−4 kV cm−1 at ν = 20 kHz, which is 5 orders of magnitude lower than in toluene. As a consequence of the very low field amplitude,
yields for the same applied voltage amplitude of Vrms = 2380 V an electric field of only Erms = 1.2 × 10−4 kV cm−1 at ν = 20 kHz, which is 5 orders of magnitude lower than in toluene. As a consequence of the very low field amplitude, 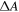 is more than 10 orders of magnitude smaller as compared to toluene, and hence not detectable as calculated in figure 6(a) using
is more than 10 orders of magnitude smaller as compared to toluene, and hence not detectable as calculated in figure 6(a) using  [17, 24]. The corresponding experimental data is shown in figure 6(b) for (8, 6) SWCNTs in 1 wt% SDS in water. No significant values for
[17, 24]. The corresponding experimental data is shown in figure 6(b) for (8, 6) SWCNTs in 1 wt% SDS in water. No significant values for 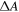 could be measured. Since Erms in water increases with ν as shown in figure S9, we simulated the dependence of
could be measured. Since Erms in water increases with ν as shown in figure S9, we simulated the dependence of  on field frequency and SWCNT length for semiconducting SWCNTs in aqueous surfactant solution. The results are shown in figure 6(a) and demonstrate that even at ν = 107 Hz, where Erms in water reaches 5.3 × 10−2 kV cm−1,
on field frequency and SWCNT length for semiconducting SWCNTs in aqueous surfactant solution. The results are shown in figure 6(a) and demonstrate that even at ν = 107 Hz, where Erms in water reaches 5.3 × 10−2 kV cm−1, 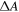 is on the order of 10−6 and therefore still too small to measure.
is on the order of 10−6 and therefore still too small to measure.
Figure 6. EFIDAS with semiconducting SWCNTs in aqueous surfactant solution. (a) Simulation of differential absorption 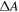 as a function of field frequency ν and nanotube length lCNT, for SWCNTs in 1 wt%-SDS in water. The horizontal dashed line indicates the field frequency used in the experiment. (b) Measurements of differential absorption spectra
as a function of field frequency ν and nanotube length lCNT, for SWCNTs in 1 wt%-SDS in water. The horizontal dashed line indicates the field frequency used in the experiment. (b) Measurements of differential absorption spectra  and absorption spectra
and absorption spectra  at zero field and at Erms = 1.2 × 10−4 kV cm−1 and ν = 20 kHz. The electric field in water is reduced by 5 orders of magnitude and
at zero field and at Erms = 1.2 × 10−4 kV cm−1 and ν = 20 kHz. The electric field in water is reduced by 5 orders of magnitude and  by 12 orders of magnitude as compared to toluene.
by 12 orders of magnitude as compared to toluene.
Download figure:
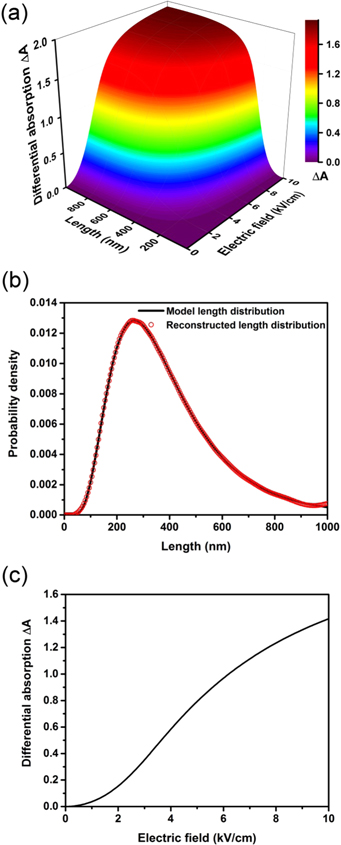
Standard image High-resolution imageFinally we evaluate the potential of EFIDAS to determine not only the chiral-index resolved mean length of a dispersion but also the (n, m)-specific length distribution 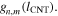 This requires measurement of
This requires measurement of 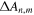 as a function of the electric field E.
as a function of the electric field E. 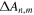 is given by
is given by  with
with  being the field
being the field 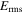 and length
and length 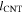 dependent (n, m)-specific differential absorption. The function
dependent (n, m)-specific differential absorption. The function 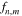 is a result of the modeling as shown in figure 7(a) and effectively maps the length distribution onto the field dependence. For demonstration purposes we use a log-normal model length distribution for
is a result of the modeling as shown in figure 7(a) and effectively maps the length distribution onto the field dependence. For demonstration purposes we use a log-normal model length distribution for  as shown in figure 7(b) and calculate
as shown in figure 7(b) and calculate 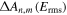 shown in figure 7(c). The length distribution is encoded in
shown in figure 7(c). The length distribution is encoded in  and can be extracted as follows. First we rewrite the above integral in vector notation
and can be extracted as follows. First we rewrite the above integral in vector notation 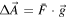 with the vectors
with the vectors  and
and  and the matrix
and the matrix  We then numerically calculate the inverse matrix
We then numerically calculate the inverse matrix 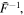 which finally allows us to reconstruct the length distribution via
which finally allows us to reconstruct the length distribution via 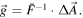 The result is shown in figure 7(b) and demonstrates that the model length distribution has indeed been nicely reconstructed by the outlined procedure. Notably, the accuracy of the approach is only limited by the size of
The result is shown in figure 7(b) and demonstrates that the model length distribution has indeed been nicely reconstructed by the outlined procedure. Notably, the accuracy of the approach is only limited by the size of 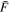 and the computational power required for calculating
and the computational power required for calculating  However, this is not a limiting factor and
However, this is not a limiting factor and  has to be calculated only once for each (n, m) SWCNT and can be further used as a look-up table. Hence we are convinced that EFIDAS allows to determine also the (n, m)-specific length distributions of SWCNTs in solution by measuring the electric-field dependent differential absorption spectrum. Testing EFIDAS with a set of monochiral dispersions should further corroborate these results.
has to be calculated only once for each (n, m) SWCNT and can be further used as a look-up table. Hence we are convinced that EFIDAS allows to determine also the (n, m)-specific length distributions of SWCNTs in solution by measuring the electric-field dependent differential absorption spectrum. Testing EFIDAS with a set of monochiral dispersions should further corroborate these results.
Figure 7. Strategy to determine (n, m)-specific length distribution of SWCNTs in dispersion with EFIDAS. (a) Simulation of the differential absorption ΔA as a function of SWCNT length lCNT and electric field Erms, for dCNT = 1.30 nm and ν = 20 kHz. (b) Log-normal model length distribution (solid line) compared to the length distribution (open symbol) reconstructed from (c) as described in the text. (c) Differential absorption versus electric field Erms for the log-normal model length distribution shown in (b).
Download figure:
Standard image High-resolution imageConclusions
EFIDS is a fast, simple and cost-effective method to measure in situ the average length of polymer-wrapped SWCNTs dispersed in toluene. Due to the spectral resolution of the method, it is possible to determine the average length of (n, m)-sub-populations in few-chiral index dispersions, which is particularly useful in the context of carbon nanotube processing as has been shown here for the case of length fractionation. The EFIDAS results are consistent with ex situ atomic force microscopy (AFM) data obtained for the same fractions, which shows that the electric-field alignment of the nanotubes can be modeled on the basis of the dielectric properties of SWCNTs and toluene without free parameters. The method cannot be applied to SWCNTs dispersed in aqueous surfactant solution due to the high conductivity and permittivity of the solvent reducing the EFIDAS signal by orders of magnitude. However by simulations we could show that the determination of the chiral-index resolved in situ length distribution of polymer-wrapped SWCNTs dispersed in toluene seems to be possible.
Materials and methods
SWCNT dispersions
Dispersions of semiconducting SWCNTs (s-SWCNTs) in toluene were prepared from pulsed laser vaporization (PLV) SWCNTs [32] using the polymer Poly(9, 9-di-n-dodecylfluorenyl-2, 7-diyl) (PODOF). The PODOF wrapped s-SWCNTs in toluene were then further length fractionated with an GPC system as described in detail in [27]. The few-chiral index dispersions contain (10, 8), (10, 9), (11, 7), (11, 9), (11, 10) and (12, 8) SWCNTs. For comparison a dispersion enriched in (8, 6) SWCNTs in water was prepared using high pressure carbon monoxide (HiPco) SWCNTs from NanoIntegris and the surfactant sodium dodecyl sulfate (SDS) by size exclusion chromatography as described in detail in [33].
Spectroscopy
EFIDAS measurements were performed in a home-made setup comprising a fiber-coupled Ocean Optics HR4000 High-Resolution Spectrometer, a fiber-coupled Mikropack DH-2000-BAL UV–vis–NIR light source, Ocean Optics collimating lenses, Thorlabs LPVIS050 linear polarizers and a Hellma 114-QS Quartz cuvette with 12.5 mm × 12.5 mm outer dimensions and 4 mm × 10 mm inner dimensions. The cuvette was loaded with its long axis parallel to the light beam, yielding an optical path length of 10 mm. Copper electrodes were attached from outside to the cuvette, parallel to the beam axis. The electrodes were biased with a Tesla generator dismantled from a commercial, low-value plasma ball. The generator has been characterized with a Testec TT HVP 15HF high-voltage probe and produces a 20 kHz signal with Vrms = 2380 V when connected to the electrodes of a toluene filled cuvette. This voltage translates into an electric field of Erms = 2.5 kV cm−1 in toluene using the expression for a capacitive voltage divider

with the thickness of the two quartz glass walls  the thickness of the toluene layer
the thickness of the toluene layer 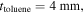 the dielectric constant of quartz glass
the dielectric constant of quartz glass 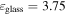 and the dielectric constant of toluene
and the dielectric constant of toluene 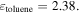 For simulations of the electric field in water
For simulations of the electric field in water 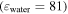 the ionic conductance has been taken into account (
the ionic conductance has been taken into account (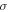 = 0.1 S m−1 for 1 wt% SDS) yielding a frequency dependence of the field amplitude as shown in figure S9. In the EFIDAS setup both polarizers were aligned parallel to the electric field axis. The spectral range of the setup is 300–1000 nm. In addition a Varian Cary 500 spectrometer was used for absorption measurements over an extended spectral range of 250–2000 nm (figure S1), without electric fields. Photoluminescence excitation maps (figure S2) were measured with a modified Bruker IFS66 FTIR spectrometer equipped with a liquid-nitrogen cooled Germanium photodiode and a monochromatized halogen lamp. The system has an excitation range of 700–950 nm and a detection range of 1250–1700 nm [34].
= 0.1 S m−1 for 1 wt% SDS) yielding a frequency dependence of the field amplitude as shown in figure S9. In the EFIDAS setup both polarizers were aligned parallel to the electric field axis. The spectral range of the setup is 300–1000 nm. In addition a Varian Cary 500 spectrometer was used for absorption measurements over an extended spectral range of 250–2000 nm (figure S1), without electric fields. Photoluminescence excitation maps (figure S2) were measured with a modified Bruker IFS66 FTIR spectrometer equipped with a liquid-nitrogen cooled Germanium photodiode and a monochromatized halogen lamp. The system has an excitation range of 700–950 nm and a detection range of 1250–1700 nm [34].
Atomic force microscopy
The diameter and length distributions of PODOF-wrapped SWCNTs were measured with a Bruker NanoScope III atomic force microscope using Mikromash silicon cantilevers in tapping mode at a resonance frequency of 320 kHz. The samples were prepared by spin-coated 0.5 μl of dispersion at 4000 rpm for 1 min onto a silicon wafer.
Calculations
Calculations were performed using Matlab R2014a software.
Acknowledgments
The authors acknowledge support from the Helmholtz Research Program Science and Technology of Nanosystems (STN). B S Flavel acknowledges support from the Deutsche Forschungsgemeinschafts Emmy Noether Program under grant number FL 834/1-1.
Conflict of interest
The authors declare no competing financial interest.