The asymptotic behaviour of subcritical dissipative quasi-geostrophic equations

In this work, we give a complete description of the asymptotic behaviour of quasi-geostrophic equations in the subcritical range . We first show that its solutions simplify asymptotically as t → ∞. More precisely, solutions behave as a particular self-similar solution normalized by the mass as t → ∞ and when the initial data belong to . On the other hand, we show that solutions with initial data in 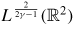decay towards zero as t → ∞ in this space. All results are obtained regardless of the size of the initial condition.