In this study, we investigate the behavior of three-dimensional parabolic–parabolic Patlak–Keller–Segel systems in the presence of ambient shear flows. Our findings demonstrate that when the total mass of the cell density is below a specific threshold, the solution remains globally regular as long as the flow is sufficiently strong. The primary difficulty in our analysis stems from the fast creation of chemical gradients due to strong shear advection.
ISSN: 1361-6544
Published jointly with the London Mathematical Society, Nonlinearity covers the interdisciplinary nature of nonlinear science, featuring topics which range from physics, mathematics and engineering through to biological sciences.
Cover credit: Christian Klein and Sergey Gavrilyuk 2024 37 045014
Siming He 2025 Nonlinearity 38 035029
Yang Liu et al 2025 Nonlinearity 38 035004
A notable feature of the elephant trunk is the pronounced wrinkling that enables its great flexibility. Here, we devise a general mathematical model that accounts for the characteristic skin wrinkles formed during morphogenesis in the elephant trunk. Using physically realistic parameters and operating within the theoretical framework of nonlinear morphoelasticity, we elucidate analytically and numerically the effect of skin thickness, relative stiffness, and differential growth on the physiological pattern of transverse wrinkles distributed along the trunk. We conclude that since the skin and muscle components have similar material properties, geometric parameters, such as curvature, play an important role. In particular, our model predicts that, in the proximal region close to the skull, where the curvature is lower, fewer wrinkles form and will form sooner than in the distal narrower region, where more wrinkles develop. Similarly, less wrinkling is found on the ventral side, which is flatter, compared to the dorsal side. In summary, the mechanical compatibility between the skin and the muscle enables them to grow seamlessly, while the wrinkled skin acts as a protective barrier that is both thicker and more flexible than the unwrinkled skin.
Helen M Byrne et al 2025 Nonlinearity 38 025022
Differential equation models are crucial to scientific processes across many disciplines, and the values of model parameters are important for analyzing the behaviour of solutions. Identifying these values is known as a parameter estimation, a type of inverse problem, which has applications in areas that include industry, finance and biomedicine. A parameter is called globally identifiable if its value can be uniquely determined from the input and output functions. Checking the global identifiability of model parameters is a useful tool when exploring the well-posedness of a given model. This problem has been intensively studied for ordinary differential equation models, where theory, several efficient algorithms and software packages have been developed. A comprehensive theory for PDEs has hitherto not been developed due to the complexity of initial and boundary conditions. Here, we provide theory and algorithms, based on differential algebra, for testing identifiability of polynomial PDE models. We showcase this approach on PDE models arising in the sciences.
Prince Romeo Mensah 2025 Nonlinearity 38 035027
We consider the Oldroyd-B model for a two-dimensional dilute corotational polymer fluid with centre-of-mass diffusion that is interacting with a one-dimensional viscoelastic shell. We show that any family of strong solutions of the system described above that is parametrized by the centre-of-mass diffusion coefficient converges, as the coefficient goes to zero, to a weak solution of a corotational polymer fluid-structure interaction system without centre-of-mass diffusion but with essentially bounded polymer number density and extra stress tensor. As a consequence, we also obtain a weak-strong uniqueness result that says that the weak solution of the latter is unique in the class of the strong solution of the former as the centre-of-mass diffusion vanishes.
Qun Wang and Ke Zhang 2025 Nonlinearity 38 035030
Treschev made the remarkable discovery that there exists formal power series describing a billiard with locally linearizable dynamics. We show that if the frequency for the linear dynamics is Diophantine, the Treschev example is 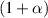 -Gevrey for some α > 0. Our proof is based on an iterative scheme that further clarifies the structure and symmetries underlying the original Treschev construction. Hopefully, our result sheds a light on the more important question of whether this example is convergent.
-Gevrey for some α > 0. Our proof is based on an iterative scheme that further clarifies the structure and symmetries underlying the original Treschev construction. Hopefully, our result sheds a light on the more important question of whether this example is convergent.
Christopher Shriver 2025 Nonlinearity 38 035023
Recent work of Barbieri and Meyerovitch has shown that, for very general spin systems indexed by sofic groups, equilibrium (i.e. pressure-maximizing) states are Gibbs. The main goal of this paper is to show that the converse fails in an interesting way: for the Ising model on a free group, the free-boundary state typically fails to be equilibrium as long as it is not the only Gibbs state. For every temperature between the uniqueness and reconstruction thresholds a typical sofic approximation gives this state finite but non-maximal pressure, and for every lower temperature the pressure is non-maximal over every sofic approximation. We also show that, for more general interactions on sofic groups, the local on average limit of Gibbs states over a sofic approximation Σ, if it exists, is a mixture of Σ-equilibrium states. We use this to show that the plus- and minus-boundary-condition Ising states are Σ-equilibrium if Σ is any sofic approximation to a free group. Combined with a result of Dembo and Montanari, this implies that these states have the same entropy over every sofic approximation.
Eduardo Muñoz-Hernández et al 2025 Nonlinearity 38 035002
We investigate traveling wave solutions for a nonlinear system of two coupled reaction-diffusion equations characterized by double degenerate diffusivity:
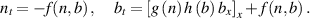
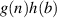 , which degenerates at n = 0 and b = 0; and the reaction term
, which degenerates at n = 0 and b = 0; and the reaction term 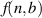 , which is positive, except for n = 0 or b = 0. Specifically, the existence of traveling wave solutions composed by a couple of strictly monotone functions for every wave speed in a closed half-line is proved, and some threshold speed estimates are given. Moreover, the regularity of the traveling wave solutions is discussed in connection with the wave speed.
, which is positive, except for n = 0 or b = 0. Specifically, the existence of traveling wave solutions composed by a couple of strictly monotone functions for every wave speed in a closed half-line is proved, and some threshold speed estimates are given. Moreover, the regularity of the traveling wave solutions is discussed in connection with the wave speed.Kristian Uldall Kristiansen and Peter Szmolyan 2025 Nonlinearity 38 025019
Any attracting, hyperbolic and proper node of a two-dimensional analytic vector-field has a unique strong-stable manifold. This manifold is analytic. The corresponding weak-stable manifolds are, on the other hand, not unique, but in the nonresonant case there is a unique weak-stable manifold that is analytic. As the system approaches a saddle-node (under parameter variation), a sequence of resonances (of increasing order) occur. In this paper, we give a detailed description of the analytic weak-stable manifolds during this process. In particular, we relate a 'flapping-mechanism', corresponding to a dramatic change of the position of the analytic weak-stable manifold as the parameter passes through the infinitely many resonances, to the lack of analyticity of the centre manifold at the saddle-node. Our work is motivated and inspired by the work of Merle, Raphaël, Rodnianski, and Szeftel, where this flapping mechanism is the crucial ingredient in the construction of 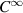 -smooth self-similar solutions of the compressible Euler equations.
-smooth self-similar solutions of the compressible Euler equations.
Chang Liu and Dejun Luo 2025 Nonlinearity 38 025009
We consider the vorticity form of 2D Navier–Stokes equations perturbed by an Ornstein–Uhlenbeck flow of transport type. Contrary to previous works where the random perturbation was interpreted as Stratonovich transport noise, here we understand the equation in a pathwise manner and show the properties of mixing and enhanced dissipation for suitable choice of the flow.
Matthieu Cadiot 2025 Nonlinearity 38 035021
In this manuscript, we present a method to prove constructively the existence and spectral stability of solitary waves in both the Whitham and the capillary–gravity Whitham equations. By employing Fourier series analysis and computer-aided techniques, we successfully approximate the Fourier multiplier operator in this equation, allowing the construction of an approximate inverse for the linearization around an approximate solution u0. Then, using a Newton–Kantorovich approach, we provide a sufficient condition under which the existence of a unique solitary wave 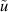 in a ball centered at u0 is obtained. The verification of such a condition is established combining analytic techniques and rigorous numerical computations. Moreover, we derive a methodology to control the spectrum of the linearization around
in a ball centered at u0 is obtained. The verification of such a condition is established combining analytic techniques and rigorous numerical computations. Moreover, we derive a methodology to control the spectrum of the linearization around 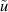 , enabling the study of spectral stability of the solution. As an illustration, we provide a (constructive) computer-assisted proof (CAP) of existence of stable solitary waves in both the case with capillary effects (T > 0) and without capillary effects (T = 0). Moreover, we provide an existence proof for a branch of solitary waves in the case T = 0 via a rigorous continuation in the wave velocity. The methodology presented in this paper can be generalized and provides a new approach for addressing the existence and spectral stability of solitary waves in nonlocal nonlinear equations. All CAPs, including the requisite codes, are accessible on GitHub at Cadiot (2023 https://github.com/matthieucadiot/WhithamSoliton.jl).
, enabling the study of spectral stability of the solution. As an illustration, we provide a (constructive) computer-assisted proof (CAP) of existence of stable solitary waves in both the case with capillary effects (T > 0) and without capillary effects (T = 0). Moreover, we provide an existence proof for a branch of solitary waves in the case T = 0 via a rigorous continuation in the wave velocity. The methodology presented in this paper can be generalized and provides a new approach for addressing the existence and spectral stability of solitary waves in nonlocal nonlinear equations. All CAPs, including the requisite codes, are accessible on GitHub at Cadiot (2023 https://github.com/matthieucadiot/WhithamSoliton.jl).
Harini Desiraju et al 2025 Nonlinearity 38 045023
We obtain rigorous large time asymptotics for the Landau–Lifshitz (LL) equation in the soliton free case by extending the nonlinear steepest descent method to genus 1 surfaces. The methods presented in this paper pave the way to a rigorous analysis of other integrable equations on the torus and enable asymptotic analysis on different regimes of the LL equation.
Carlos M Mora 2025 Nonlinearity 38 045021
We develop a system of non-linear stochastic evolution equations that describes the continuous measurements of quantum systems with mixed initial state. We address quantum systems with unbounded Hamiltonians and unbounded interaction operators. Using arguments of the theory of quantum measurements we derive a system of stochastic interacting wave functions (SIWFs for short) that models the continuous monitoring of quantum systems. We prove the existence and uniqueness of the solution to this system under conditions general enough for the applications. We obtain that the mixed state generated by the SIWF at any time does not depend on the initial state, and satisfies the diffusive stochastic quantum master equation, which is also known as Belavkin equation. We present two physical examples. In one, the SIWF becomes a system of non-linear stochastic partial differential equations. In the other, we deal with a model of a circuit quantum electrodynamics.
Julian Newman et al 2025 Nonlinearity 38 045022
Various definitions of an attractor for a nonlinear dynamical system have been proposed. These use various assumptions on the set of initial conditions that should converge (the basin), and various notions of convergence. A weak assumption on the basin is the measure attractor of Milnor, which requires that the basin has positive measure. A weak assumption of the notion of convergence is the statistical attractor due to Ilyashenko, which requires that limiting to the attractor occurs on a set of future times of full density. We point out that many examples of statistical attractors actually satisfy a stronger definition which we call a bounded-return-time attractor, and we investigate such attractors. We also give an improved definition for the notion of pullback measure attraction. This was originally developed to understand attractors in nonautonomous systems, but we note here that it is helpful for understanding convergence towards statistical attractors in the autonomous setting. We investigate implications between all these different notions of attractors. We also investigate which of these notions are fulfilled by a hyperbolic fixed point with a homoclinic loop.
S van Golden et al 2025 Nonlinearity 38 045020
For countably infinite IFSs on 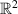 consisting of affine contractions with diagonal linear parts, we give conditions under which the affinity dimension is an upper bound for the Hausdorff dimension and a lower bound for the lower box-counting dimension. Moreover, we identify a family of countably infinite IFSs for which the Hausdorff and the affinity dimension are equal, and which have full dimension spectrum. The corresponding self-affine sets are related to restricted digit sets for signed Lüroth expansions.
consisting of affine contractions with diagonal linear parts, we give conditions under which the affinity dimension is an upper bound for the Hausdorff dimension and a lower bound for the lower box-counting dimension. Moreover, we identify a family of countably infinite IFSs for which the Hausdorff and the affinity dimension are equal, and which have full dimension spectrum. The corresponding self-affine sets are related to restricted digit sets for signed Lüroth expansions.
Nathanaël Boutillon and Luca Rossi 2025 Nonlinearity 38 045019
We consider a reaction-diffusion model for a population structured in phenotype. We assume that the population lives in a heterogeneous periodic environment, so that a given phenotypic trait may be more or less fit according to the spatial location. The model features spatial mobility of individuals as well as mutation. We first prove the well-posedness of the model. Next, we derive a criterion for the persistence of the population which involves the generalised principal eigenvalue associated with the linearised elliptic operator. This notion allows us to handle the possible lack of coercivity of the operator. We then obtain a monotonicity result for the generalised principal eigenvalue, in terms of the frequency of spatial fluctuations of the environment and in terms of the spatial diffusivity. We deduce that the more heterogeneous is the environment, or the higher is the mobility of individuals, the harder is the persistence for the species. This work lays the mathematical foundation to investigate some other optimisation problems for the environment to make persistence as hard or as easy as possible, which will be addressed in the forthcoming companion paper.
Ryan Goh and Arnd Scheel 2023 Nonlinearity 36 R1
Pattern forming systems allow for a wealth of states, where wavelengths and orientation of patterns varies and defects disrupt patches of monocrystalline regions. Growth of patterns has long been recognized as a strong selection mechanism. We present here recent and new results on the selection of patterns in situations where the pattern-forming region expands in time. The wealth of phenomena is roughly organised in bifurcation diagrams that depict wavenumbers of selected crystalline states as functions of growth rates. We show how a broad set of mathematical and numerical tools can help shed light into the complexity of this selection process.
Ali Tahzibi 2021 Nonlinearity 34 R75
In this survey we recall basic notions of disintegration of measures and entropy along unstable laminations. We review some roles of unstable entropy in smooth ergodic theory including the so-called invariance principle, Margulis construction of measures of maximal entropy, physical measures and rigidity. We also give some new examples and pose some open problems.
Thomas Bothner 2021 Nonlinearity 34 R1
This article is firstly a historic review of the theory of Riemann–Hilbert problems with particular emphasis placed on their original appearance in the context of Hilbert's 21st problem and Plemelj's work associated with it. The secondary purpose of this note is to invite a new generation of mathematicians to the fascinating world of Riemann–Hilbert techniques and their modern appearances in nonlinear mathematical physics. We set out to achieve this goal with six examples, including a new proof of the integro-differential Painlevé-II formula of Amir et al (2011 Commun. Pure Appl. Math.64 466–537) that enters in the description of the Kardar–Parisi–Zhang crossover distribution. Parts of this text are based on the author's Szegő prize lecture at the 15th International Symposium on Orthogonal Polynomials, Special Functions and Applications (OPSFA) in Hagenberg, Austria.
Valerio Lucarini and Tamás Bódai 2020 Nonlinearity 33 R59
For a wide range of values of the intensity of the incoming solar radiation, the Earth features at least two attracting states, which correspond to competing climates. The warm climate is analogous to the present one; the snowball climate features global glaciation and conditions that can hardly support life forms. Paleoclimatic evidences suggest that in the past our planet flipped between these two states. The main physical mechanism responsible for such an instability is the ice-albedo feedback. In a previous work, we defined the Melancholia states that sit between the two climates. Such states are embedded in the boundaries between the two basins of attraction and feature extensive glaciation down to relatively low latitudes. Here, we explore the global stability properties of the system by introducing random perturbations as modulations to the intensity of the incoming solar radiation. We observe noise-induced transitions between the competing basins of attraction. In the weak-noise limit, large deviation laws define the invariant measure, the statistics of escape times, and typical escape paths called instantons. By constructing the instantons empirically, we show that the Melancholia states are the gateways for the noise-induced transitions. In the region of multistability, in the zero-noise limit, the measure is supported only on one of the competing attractors. For low (high) values of the solar irradiance, the limit measure is the snowball (warm) climate. The changeover between the two regimes corresponds to a first-order phase transition in the system. The framework we propose seems of general relevance for the study of complex multistable systems. Finally, we put forward a new method for constructing Melancholia states from direct numerical simulations, which provides a possible alternative with respect to the edge-tracking algorithm.
D Lannes 2020 Nonlinearity 33 R1
We review here the derivation of many of the most important models that appear in the literature (mainly in coastal oceanography) for the description of waves in shallow water. We show that these models can be obtained using various asymptotic expansions of the 'turbulent' and non-hydrostatic terms that appear in the equations that result from the vertical integration of the free surface Euler equations. Among these models are the well-known nonlinear shallow water (NSW), Boussinesq and Serre–Green–Naghdi (SGN) equations for which we review several pending open problems. More recent models such as the multi-layer NSW or SGN systems, as well as the Isobe–Kakinuma equations are also reviewed under a unified formalism that should simplify comparisons. We also comment on the scalar versions of the various shallow water systems which can be used to describe unidirectional waves in horizontal dimension d = 1; among them are the KdV, BBM, Camassa–Holm and Whitham equations. Finally, we show how to take vorticity effects into account in shallow water modeling, with specific focus on the behavior of the turbulent terms. As examples of challenges that go beyond the present scope of mathematical justification, we review recent works using shallow water models with vorticity to describe wave breaking, and also derive models for the propagation of shallow water waves over strong currents.
Harini Desiraju et al 2025 Nonlinearity 38 045023
We obtain rigorous large time asymptotics for the Landau–Lifshitz (LL) equation in the soliton free case by extending the nonlinear steepest descent method to genus 1 surfaces. The methods presented in this paper pave the way to a rigorous analysis of other integrable equations on the torus and enable asymptotic analysis on different regimes of the LL equation.
Carlos M Mora 2025 Nonlinearity 38 045021
We develop a system of non-linear stochastic evolution equations that describes the continuous measurements of quantum systems with mixed initial state. We address quantum systems with unbounded Hamiltonians and unbounded interaction operators. Using arguments of the theory of quantum measurements we derive a system of stochastic interacting wave functions (SIWFs for short) that models the continuous monitoring of quantum systems. We prove the existence and uniqueness of the solution to this system under conditions general enough for the applications. We obtain that the mixed state generated by the SIWF at any time does not depend on the initial state, and satisfies the diffusive stochastic quantum master equation, which is also known as Belavkin equation. We present two physical examples. In one, the SIWF becomes a system of non-linear stochastic partial differential equations. In the other, we deal with a model of a circuit quantum electrodynamics.
Julian Newman et al 2025 Nonlinearity 38 045022
Various definitions of an attractor for a nonlinear dynamical system have been proposed. These use various assumptions on the set of initial conditions that should converge (the basin), and various notions of convergence. A weak assumption on the basin is the measure attractor of Milnor, which requires that the basin has positive measure. A weak assumption of the notion of convergence is the statistical attractor due to Ilyashenko, which requires that limiting to the attractor occurs on a set of future times of full density. We point out that many examples of statistical attractors actually satisfy a stronger definition which we call a bounded-return-time attractor, and we investigate such attractors. We also give an improved definition for the notion of pullback measure attraction. This was originally developed to understand attractors in nonautonomous systems, but we note here that it is helpful for understanding convergence towards statistical attractors in the autonomous setting. We investigate implications between all these different notions of attractors. We also investigate which of these notions are fulfilled by a hyperbolic fixed point with a homoclinic loop.
S van Golden et al 2025 Nonlinearity 38 045020
For countably infinite IFSs on 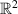 consisting of affine contractions with diagonal linear parts, we give conditions under which the affinity dimension is an upper bound for the Hausdorff dimension and a lower bound for the lower box-counting dimension. Moreover, we identify a family of countably infinite IFSs for which the Hausdorff and the affinity dimension are equal, and which have full dimension spectrum. The corresponding self-affine sets are related to restricted digit sets for signed Lüroth expansions.
consisting of affine contractions with diagonal linear parts, we give conditions under which the affinity dimension is an upper bound for the Hausdorff dimension and a lower bound for the lower box-counting dimension. Moreover, we identify a family of countably infinite IFSs for which the Hausdorff and the affinity dimension are equal, and which have full dimension spectrum. The corresponding self-affine sets are related to restricted digit sets for signed Lüroth expansions.
Matthieu Cadiot and Dominic Blanco 2025 Nonlinearity 38 045016
In this article, we present a comprehensive framework for constructing smooth, localized solutions in systems of semi-linear partial differential equations, with a particular emphasis to the Gray–Scott model. Specifically, we construct a natural Hilbert space 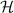 for the study of systems of autonomous semi-linear PDEs, on which products and differential operators are well-defined. Then, given an approximate solution u0, we derive a Newton–Kantorovich approach based on the construction of an approximate inverse of the linearization around u0. In particular, we derive a condition under which we prove the existence of a unique solution in a neighborhood of u0. Such a condition can be verified thanks to the explicit computation of different upper bounds, for which analytical details are presented. Furthermore, we provide an extra condition under which localized patterns are proven to be the limit of an unbounded branch of (spatially) periodic solutions as the period tends to infinity. We then demonstrate our approach by proving (constructively) the existence of four different localized patterns in the 2D Gray–Scott model. In addition, these solutions are proven to satisfy the D4-symmetry. That is, the symmetry of the square. The algorithmic details to perform the computer-assisted proofs are available on GitHub (2024 LocalizedPatternsGS.jl https://github.com/dominicblanco/LocalizedPatternsGS.jl).
for the study of systems of autonomous semi-linear PDEs, on which products and differential operators are well-defined. Then, given an approximate solution u0, we derive a Newton–Kantorovich approach based on the construction of an approximate inverse of the linearization around u0. In particular, we derive a condition under which we prove the existence of a unique solution in a neighborhood of u0. Such a condition can be verified thanks to the explicit computation of different upper bounds, for which analytical details are presented. Furthermore, we provide an extra condition under which localized patterns are proven to be the limit of an unbounded branch of (spatially) periodic solutions as the period tends to infinity. We then demonstrate our approach by proving (constructively) the existence of four different localized patterns in the 2D Gray–Scott model. In addition, these solutions are proven to satisfy the D4-symmetry. That is, the symmetry of the square. The algorithmic details to perform the computer-assisted proofs are available on GitHub (2024 LocalizedPatternsGS.jl https://github.com/dominicblanco/LocalizedPatternsGS.jl).
D A Rand and M Sáez 2025 Nonlinearity 38 045015
We consider generic families Xθ of smooth dynamical systems depending on parameters 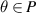 where P is a 2-dimensional simply connected domain and assume that each Xθ only has a finite number of restpoints and periodic orbits. We prove that if over the boundary of P there is a S or Z shaped bifurcation graph containing two opposing fold bifurcation points while over the rest of the boundary there are no other bifurcation points, then, if there is no fold–Hopf bifurcation in P, there is a set of bifurcation curves in P that contain an odd number of cusps. In particular, there is at least one codimension 2 bifurcation point in the interior of P.
where P is a 2-dimensional simply connected domain and assume that each Xθ only has a finite number of restpoints and periodic orbits. We prove that if over the boundary of P there is a S or Z shaped bifurcation graph containing two opposing fold bifurcation points while over the rest of the boundary there are no other bifurcation points, then, if there is no fold–Hopf bifurcation in P, there is a set of bifurcation curves in P that contain an odd number of cusps. In particular, there is at least one codimension 2 bifurcation point in the interior of P.
Andrea Aspri et al 2025 Nonlinearity 38 045014
In this paper, we study the monodomain model of cardiac electrophysiology, which is widely used to describe the propagation of electrical signals in cardiac tissue. The forward problem, described by a reaction–diffusion equation coupled with an ordinary differential equation in a domain containing a perfectly insulating region, is first analysed to establish its well-posedness under standard assumptions on the conductivity and ionic current terms. We then investigate the inverse problem of identifying perfectly insulating regions within the cardiac tissue, which serve as mathematical representations of ischemic areas. These regions are characterised by a complete lack of electrical conductivity, impacting the propagation of electrical signals. We prove that the geometry and location of these insulating regions can be uniquely determined using only partial boundary measurements of the transmembrane potential. Our approach combines tools from elliptic and parabolic PDE theory, Carleman estimates, and the analysis of unique continuation properties. These results contribute to the theoretical understanding of diagnostic methods in cardiology.
Gui-Qiang G Chen et al 2025 Nonlinearity 38 045013
We establish the optimal convergence rate of the hypersonic similarity for two-dimensional steady potential flows with large data past a straight wedge in the 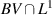 framework, provided that the total variation of the large data multiplied by
framework, provided that the total variation of the large data multiplied by 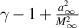 is uniformly bounded with respect to the adiabatic exponent γ > 1, the Mach number
is uniformly bounded with respect to the adiabatic exponent γ > 1, the Mach number 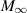 of the incoming steady flow, and the hypersonic similarity parameter
of the incoming steady flow, and the hypersonic similarity parameter 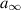 . Our main approach in this paper is first to establish the well-posedness and the Lipschitz continuous map
. Our main approach in this paper is first to establish the well-posedness and the Lipschitz continuous map 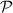 that has the properties similar to the Standard Riemann Semigroup of the initial-boundary value problem for the isothermal hypersonic small disturbance equations with large data, and then to compare the Riemann solutions between two systems with boundary locally case by case. Based on them, we derive the global L1–estimate between the two solutions by employing the Lipschitz continuous map
that has the properties similar to the Standard Riemann Semigroup of the initial-boundary value problem for the isothermal hypersonic small disturbance equations with large data, and then to compare the Riemann solutions between two systems with boundary locally case by case. Based on them, we derive the global L1–estimate between the two solutions by employing the Lipschitz continuous map 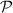 and the local L1–estimates. We further construct an example to show that the convergence rate is optimal.
and the local L1–estimates. We further construct an example to show that the convergence rate is optimal.
Gianni Arioli and J D Mireles James 2025 Nonlinearity 38 045010
The goal of this paper it to prove existence theorems for one parameter families (branches) of ejection-collision orbits in the planar circular restricted three body problem (CRTBP), and to study some of bifurcations of these branches. The CRTBP considers the dynamics of an infinitesimal particle moving in the gravitational field of two massive primary bodies. The motion of the primaries assumed to be circular, and we study ejection–collision orbits where the infinitesimal body is ejected from one primary and collides with the other (as opposed to more local ejections–collisions where the infinitesimal body collides with a single primary body in both forward and backward time). We consider branches of ejection–collision orbits which are (i) parameterized by the Jacobi integral (energy like quantity conserved by the CRTBP) with the masses of the primaries fixed, and (ii) parameterized by the mass ratio of the primary bodies with energy fixed. The method of proof is constructive and computer assisted, hence can be applied in non-perturbative settings and (potentially) to other conservative systems of differential equations. The main requirement is that the system should admit a change of coordinates which regularizes the collision singularities. In the planar CRTBP, the necessary regularization is provided by the classical Levi–Civita transformation.
Federica Dragoni et al 2025 Nonlinearity 38 045009
We show that the square of Carnot–Carathéodory distance from the origin, in step 2 Carnot groups, enjoys the horizontal semiconcavity (h-semiconcavity) everywhere in the group including the origin. We first give a proof in the case of ideal Carnot groups, based on the simple group structure as well as estimates for the Euclidean semiconcavity. Our proof of the general result involves more geometric properties of step 2 Carnot groups. We further apply our h-semiconcavity result to show h-semiconcavity of the viscosity solutions to a class of non-coercive evolutive Hamilton–Jacobi equations by using the Hopf–Lax formula associated to the Carnot–Carathéodory metric.


