Abstract
It is shown that calculation of the momentum Fisher information of the quasi-one-dimensional hydrogen atom recently presented by Saha et al (2017 Eur. J. Phys. 38 025103) is wrong. A correct derivation is provided and its didactical advantages and scientific significances are highlighted.
Export citation and abstract BibTeX RIS
Recently, a calculation of the position Iρ and momentum Iγ Fisher information was presented for (i) a linear harmonic oscillator, (ii) a quasi-one-dimensional hydrogen atom and (iii) an infinite potential well [1]. By general definition [2, 3], these quantum-information measures for arbitrary one-dimensional (1D) structures are defined as
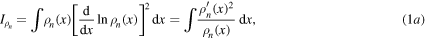
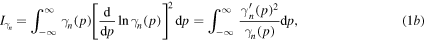
where a position integration is carried out over all available interval x and positive integer index n counts all possible quantum states. In these equations,  and
and  are position and momentum probability density, respectively:
are position and momentum probability density, respectively:


and corresponding waveforms  and
and  are related through the Fourier transformation:
are related through the Fourier transformation:
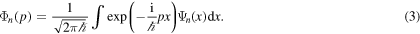
Both of them satisfy orthonormality conditions:


where  is a Kronecker delta,
is a Kronecker delta,  . Real position wave function
. Real position wave function  and associated eigen energy En are found from the 1D Schrödinger equation:
and associated eigen energy En are found from the 1D Schrödinger equation:
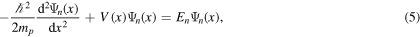
with mp being a mass of the particle and V(x) being an external potential.
First, we point out that for the infinite potential well of the width a the Fisher informations were calculated before [4] where the position component was evaluated directly from equation (1a) whereas for finding  an elegant and didactically instructive method was used; namely, since for this geometry both
an elegant and didactically instructive method was used; namely, since for this geometry both  and
and  are real, the integrand in equation (1b) becomes
are real, the integrand in equation (1b) becomes ![$4{[{{\rm{\Phi }}}_{n}^{\prime }(p)]}^{2}$](https://content.cld.iop.org/journals/0143-0807/38/3/038001/revision1/ejpaa62cdieqn11.gif) , and using the reciprocity between position and momentum spaces, equation (3), one replaces infinite p integration by the finite x one:
, and using the reciprocity between position and momentum spaces, equation (3), one replaces infinite p integration by the finite x one:

Turning to the discussion of the quasi-1D hydrogen atom, it has to be noted that a problem of the quantum motion along the whole x axis,  , in the potential
, in the potential  , despite its long history, still remains (owing to the strong singularity at the origin and concomitant difficulty of matching right and left solutions at x = 0) a topic of debate and controversy, see, e.g., [5, 6] and literature cited therein. A situation is somewhat simplified when one confines the motion only to the right half space terminated at x = 0 by the infinite barrier. Accordingly, let us consider solutions of the Schrödinger equation (5) with the potential [1]
, despite its long history, still remains (owing to the strong singularity at the origin and concomitant difficulty of matching right and left solutions at x = 0) a topic of debate and controversy, see, e.g., [5, 6] and literature cited therein. A situation is somewhat simplified when one confines the motion only to the right half space terminated at x = 0 by the infinite barrier. Accordingly, let us consider solutions of the Schrödinger equation (5) with the potential [1]

 . Upon introducing Coulomb units where energies and distances are measured in terms of
. Upon introducing Coulomb units where energies and distances are measured in terms of  and
and  , respectively [7], and momenta—in units of
, respectively [7], and momenta—in units of  , one arrives at the differential equation
, one arrives at the differential equation
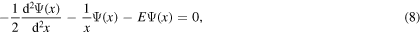
whose general solution for the negative energies,  , corresponding to the bound states, reads:
, corresponding to the bound states, reads:
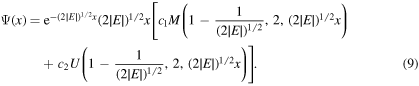
Here,  and
and  are Kummer, or confluent hypergeometric, functions (we follow the notation adopted in [8]), and c1 and c2 are normalisation constants. Physically, this mathematical solution vanishes at the origin and, since the second item in the square brackets of the right-hand side of equation (9) diverges at
are Kummer, or confluent hypergeometric, functions (we follow the notation adopted in [8]), and c1 and c2 are normalisation constants. Physically, this mathematical solution vanishes at the origin and, since the second item in the square brackets of the right-hand side of equation (9) diverges at  [8], it has to be neglected,
[8], it has to be neglected,  . Remaining part must decay sufficiently fast at infinity. From the properties of the Kummer function
. Remaining part must decay sufficiently fast at infinity. From the properties of the Kummer function  [8] it follows that it is possible only when its first parameter is equal to the nonpositive integer what immediately leads to the energy spectrum coinciding with the 3D hydrogen atom [7]
[8] it follows that it is possible only when its first parameter is equal to the nonpositive integer what immediately leads to the energy spectrum coinciding with the 3D hydrogen atom [7]

whereas the corresponding waveform simplifies to

with  ,
,  , being a generalised Laguerre polynomial [8]. Figure 1 shows waveforms of the first four levels. Didactically, a representation of the solution in the form of the Laguerre polynomials is much more advantageous compared to that of the confluent hypergeometric functions, equation (16) in [1]; in particular, utilising properties of the Laguerre polynomials (see equation 2.19.14.18 in [9]), one instantly confirms that equation (11) does satisfy the orthonormality condition, equation (4a), for
, being a generalised Laguerre polynomial [8]. Figure 1 shows waveforms of the first four levels. Didactically, a representation of the solution in the form of the Laguerre polynomials is much more advantageous compared to that of the confluent hypergeometric functions, equation (16) in [1]; in particular, utilising properties of the Laguerre polynomials (see equation 2.19.14.18 in [9]), one instantly confirms that equation (11) does satisfy the orthonormality condition, equation (4a), for  .1
Moreover, the form of solution from equation (11) allows an instructive calculation from equation (3) of the momentum waveform. For doing this, one recalls Rodrigues formula for Laguerre polynomials [8]:
.1
Moreover, the form of solution from equation (11) allows an instructive calculation from equation (3) of the momentum waveform. For doing this, one recalls Rodrigues formula for Laguerre polynomials [8]:

Then,  becomes:
becomes:
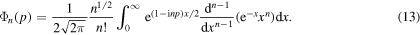
Successive  integrations by parts simplify this to:
integrations by parts simplify this to:
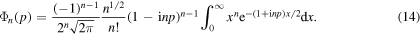
An elementary deformation  of the integration contour in this equation yields ultimately:
of the integration contour in this equation yields ultimately:
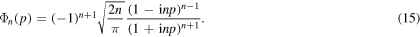
Observe that this complex solution is completely different from the real one provided by equation (17) from [1]. With the help of residue theorem applied to calculation of the integrals with infinite limits [10], it is elementary to check that the set from equation (15) does obey equation (4b), as expected. The dependencies of the real and imaginary parts of the waveforms  on the momentum are shown in figure 2. It is seen that the number and amplitude of the oscillations increase for the higher quantum indices n.
on the momentum are shown in figure 2. It is seen that the number and amplitude of the oscillations increase for the higher quantum indices n.
Figure 1. Position wave functions  where solid line denotes ground state, dotted curve—first excited level, dashed line depicts the orbital with the quantum index n = 3, and the dash–dotted line is for the state with n = 4.
where solid line denotes ground state, dotted curve—first excited level, dashed line depicts the orbital with the quantum index n = 3, and the dash–dotted line is for the state with n = 4.
Download figure:
Standard image High-resolution imageFigure 2. Momentum wave functions  where each panel corresponds to the quantum level with the number depicted inside it. Solid (dash) lines denote real (imaginary) components. Since real (imaginary) part of
where each panel corresponds to the quantum level with the number depicted inside it. Solid (dash) lines denote real (imaginary) components. Since real (imaginary) part of  is symmetric (antisymmetric) function of momentum, the dependencies for nonnegative p only are shown.
is symmetric (antisymmetric) function of momentum, the dependencies for nonnegative p only are shown.
Download figure:
Standard image High-resolution imageKnowledge of the functions  and
and  and, accordingly, of the corresponding densities
and, accordingly, of the corresponding densities


paves the way to calculating the associated Fisher informations. Dropping quite simple intermediate computations (which in the case of the position component  rely on the properties of the Laguerre polynomials [8, 9] while for its momentum counterpart
rely on the properties of the Laguerre polynomials [8, 9] while for its momentum counterpart  elementary properties of the integrals of the product of the power and algebraic functions are employed), the ultimate results are given as
elementary properties of the integrals of the product of the power and algebraic functions are employed), the ultimate results are given as


what leads to the index independent product

Note that position Fisher information whose expression, equation (17a), does coincide with its counterpart from [1] is proportional to the absolute value of energy what is its general property while the momentum component is just the inverse of  . As a result, the product of the two informations stays the same for all levels. This n independence singles out the hydrogen atom from other two structures studied in [1] for which
. As a result, the product of the two informations stays the same for all levels. This n independence singles out the hydrogen atom from other two structures studied in [1] for which  is a quadratic function of the quantum index.
is a quadratic function of the quantum index.
Footnotes
- 1







